Advertisements
Advertisements
Question
In three line segments OA, OB, and OC, points L, M, N respectively are so chosen that LM || AB and MN || BC but neither of L, M, N nor of A, B, C are collinear. Show that LN ||AC.
Solution
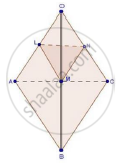
We have,
LM || AB and MN || BC
Therefore, by basic proportionality theorem,
We have,
`"QL"/"AL"="OM"/"MB"` ..........(i)
and, `"ON"/"NC"="OM"/"MB"` ..........(ii)
Comparing equation (i) and equation (ii), we get,
`"ON"/"AL"="ON"/"NC"`
Thus, LN divides sides OA and OC of ΔOAC in the same ratio. Therefore, by the converse of basic proportionality theorem,
we have, LN || AC
APPEARS IN
RELATED QUESTIONS
D and E are points on the sides AB and AC respectively of a ΔABC such that DE║BC.
If AD = 3.6cm, AB = 10cm and AE = 4.5cm, find EC and AC.
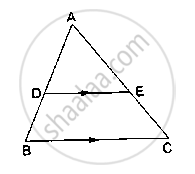
D and E are points on the sides AB and AC respectively of a ΔABC such that DE║BC.
If AB = 13.3cm, AC = 11.9cm and EC = 5.1cm, find AD.
In the adjoining figure, ABC is a triangle in which AB = AC. IF D and E are points on AB and AC respectively such that AD = AE, show that the points B, C, E and D are concyclic.

ΔABC is am equilateral triangle of side 2a units. Find each of its altitudes.
Find the height of an equilateral triangle of side 12cm.
Find the length of each side of a rhombus whose diagonals are 24cm and 10cm long.
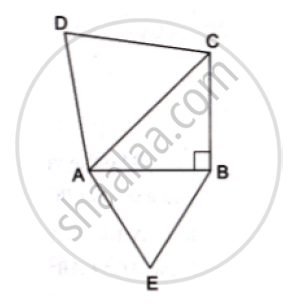
ABC is an isosceles triangle, right-angled at B. Similar triangles ACD and ABE are constructed on sides AC and AB. Find the ratio between the areas of ΔABE and ΔACD.
An aeroplane leaves an airport and flies due north at a speed of 1000km per hour. At the same time, another aeroplane leaves the same airport and flies due west at a speed of 1200 km per hour. How far apart will be the two planes after` 1 1/2` hours?
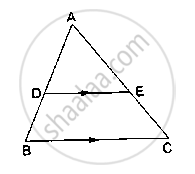
In the given figure, ABC is a triangle in which DE||BC. If AD = x, DB = x – 2, AE = x + 2 and EC = x – 1, then find the value of x.
State and prove Basic Proportionality theorem.
