Advertisements
Advertisements
Question
In triangle ABC, the medians BP and CQ are produced up to points M and N respectively such that BP = PM and CQ = QN. Prove that:
- M, A, and N are collinear.
- A is the mid-point of MN.
Solution
The figure is shown below
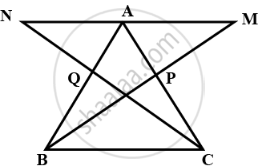
(i) In ΔAQN & ΔBQC
AQ = QB (Given)
∠AQN = ∠BQC
QN = QC
∴ ΔAQN ≅ ΔBQC ...[ by SAS ]
∴ ∠QAN = ∠QBC ...(1)
And BC = AN ……(2)
Similarly, ΔAPM ≅ ΔCPB .....[by SAS]
∠PAM = ∠PCB ...(3) [by CPTC]
And BC = AM ….( 4 )
Now In ΔABC,
∠ABC + ∠ACB + ∠BAC = 180°
∠QAN + ∠PAM + ∠BAC = 180° ...[ (1), (2) we get ]
Therefore M, A, N are collinear.
(ii) From (3) and (4) MA = NA
Hence A is the midpoint of MN.
APPEARS IN
RELATED QUESTIONS
ABCD is a rhombus, EABF is a straight line such that EA = AB = BF. Prove that ED and FC when produced meet at right angles
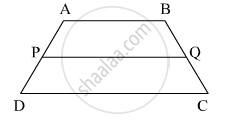
In the Figure, `square`ABCD is a trapezium. AB || DC. Points P and Q are midpoints of seg AD and seg BC respectively. Then prove that, PQ || AB and PQ = `1/2 ("AB" + "DC")`.
In the given figure, M is mid-point of AB and DE, whereas N is mid-point of BC and DF.
Show that: EF = AC.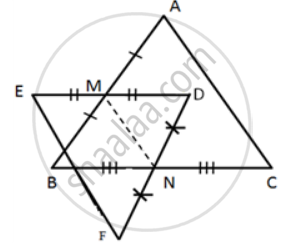
In a triangle ABC, AD is a median and E is mid-point of median AD. A line through B and E meets AC at point F.
Prove that: AC = 3AF.
If the quadrilateral formed by joining the mid-points of the adjacent sides of quadrilateral ABCD is a rectangle,
show that the diagonals AC and BD intersect at the right angle.
In ΔABC, D is the mid-point of AB and E is the mid-point of BC.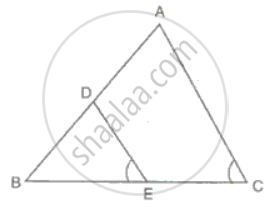
Calculate:
(i) DE, if AC = 8.6 cm
(ii) ∠DEB, if ∠ACB = 72°
In ΔABC, D, E and F are the midpoints of AB, BC and AC.
Show that AE and DF bisect each other.
In a parallelogram ABCD, E and F are the midpoints of the sides AB and CD respectively. The line segments AF and BF meet the line segments DE and CE at points G and H respectively Prove that: ΔGEA ≅ ΔGFD
In ΔABC, D and E are the midpoints of the sides AB and AC respectively. F is any point on the side BC. If DE intersects AF at P show that DP = PE.
The quadrilateral formed by joining the mid-points of the sides of a quadrilateral PQRS, taken in order, is a rhombus, if ______.
