Advertisements
Advertisements
Question
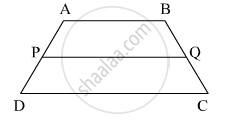
In the Figure, `square`ABCD is a trapezium. AB || DC. Points P and Q are midpoints of seg AD and seg BC respectively. Then prove that, PQ || AB and PQ = `1/2 ("AB" + "DC")`.
Solution
Given: `square`ABCD is a trapezium.
To prove: PQ || AB and PQ = `1/2`(AB + DC)
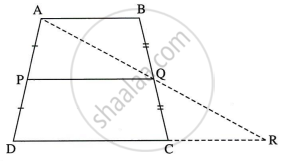
Construction: Extend line AQ in such a way that, on extending side DC, intersect it at point R.
Proof:
seg AB || seg DC ...(Given)
and seg BC is their transversal.
∴ ∠ABC ≅ ∠RCB ...(Alternate angles)
∴ ∠ABQ ≅ ∠RCQ ...(i) ...(B-Q-C)
In ∆ABQ and ∆RCQ,
∠ABQ ≅∠RCQ ...[From (i)]
seg BQ ≅ seg CQ ...(Q is the midpoint of seg BC)
∠BQA ≅ ∠CQR ...(Vertically opposite angles)
∴ ∆ABQ ≅ ∆RCQ ...(ASA test)
seg AB ≅ seg CR ...(c.s.c.t.) ...(ii)
seg AQ ≅ seg RQ ...(c.s.c.t.) ...(iii)
In ∆ADR,
Point P is the midpoint of line AD. ...(Given)
Point Q is the midpoint of line AR. ...[From (iii)]
∴ seg PQ || side DR ...(Midpoint Theorem)
∴ seg PQ || side DC ...(iv) ...(D-C-R)
∴ side AB || side DC ...(v) ...(Given)
∴ seg PQ || side AB ...[From (iv) and (v)]
PQ = `1/2` DR ...(Midpoint Theorem)
= `1/2` (DC + CR)
= `1/2` (DC + AB) ...[From (ii)]
∴ PQ = `1/2` (AB + DC)
APPEARS IN
RELATED QUESTIONS
ABCD is a square E, F, G and H are points on AB, BC, CD and DA respectively, such that AE = BF = CG = DH. Prove that EFGH is a square.
BM and CN are perpendiculars to a line passing through the vertex A of a triangle ABC. If
L is the mid-point of BC, prove that LM = LN.
In triangle ABC, M is mid-point of AB and a straight line through M and parallel to BC cuts AC in N. Find the lengths of AN and MN if Bc = 7 cm and Ac = 5 cm.
D, E, and F are the mid-points of the sides AB, BC and CA of an isosceles ΔABC in which AB = BC.
Prove that ΔDEF is also isosceles.
The figure, given below, shows a trapezium ABCD. M and N are the mid-point of the non-parallel sides AD and BC respectively. Find:

- MN, if AB = 11 cm and DC = 8 cm.
- AB, if DC = 20 cm and MN = 27 cm.
- DC, if MN = 15 cm and AB = 23 cm.
The diagonals of a quadrilateral intersect at right angles. Prove that the figure obtained by joining the mid-points of the adjacent sides of the quadrilateral is rectangle.
ABCD is a parallelogram.E is the mid-point of CD and P is a point on AC such that PC = `(1)/(4)"AC"`. EP produced meets BC at F. Prove that: 2EF = BD.
The diagonals AC and BD of a quadrilateral ABCD intersect at right angles. Prove that the quadrilateral formed by joining the midpoints of quadrilateral ABCD is a rectangle.
In the given figure, T is the midpoint of QR. Side PR of ΔPQR is extended to S such that R divides PS in the ratio 2:1. TV and WR are drawn parallel to PQ. Prove that T divides SU in the ratio 2:1 and WR = `(1)/(4)"PQ"`.
Prove that the line joining the mid-points of the diagonals of a trapezium is parallel to the parallel sides of the trapezium.
