Advertisements
Advertisements
Question
In ∆PQR, right-angled at Q, PQ = 3 cm and PR = 6 cm. Determine ∠P and ∠R.
Solution
We are given the following information in the form of the triangle
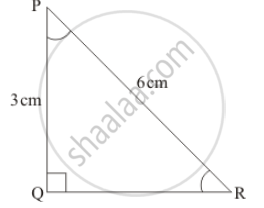
To find ∠P and ∠R
Now in ΔPQR
`cos P = (PQ)/(PR)`
`cos P = 3/6` .....(1)
`= 1/2 `
Now we know that
`cos 60^@ = 1/2` ....(2)
Now by comparing equation (1) and (2)
We get,
`∠P = 60^@ ....(3)`
Now we have
`sin P =(QR)/(PR)`
`sin 60^@= (QR)/6`
Now we know that
`sin 60^@ = sqrt3/2`
Therefore,
`sqrt3/2 = (QR)/6`
Now by cross multiplying
We get
`6 xx sqrt3 = 2 xx QR`
`=> 6sqrt3 = 2QR`
`=> QR = (6sqrt3)/2`
`=> QR = 3sqrt3`
Therefore
`QR = 3sqrt3 cm` .....(4)
Now we know that
`cos R = (QR)/(PR)`
`cos R = (3sqrt3)/2`
`=> cos R = sqrt3/2` ....(5)
Now we know,
`cos 30^@ = sqrt3/2` ....(6)
Now by comparing equation (5) and (6)
We get,
∠R = 30° ...(7)
Hence from equation (3) and (7)
`∠P = 60^@ and ∠R = 30^@`
APPEARS IN
RELATED QUESTIONS
If A = B = 60°, verify that cos (A − B) = cos A cos B + sin A sin B
Evaluate:
`2cos^2 60^0+3 sin^2 45^0 - 3 sin^2 30^0 + 2 cos^2 90 ^0`
In the adjoining figure, ΔABC is right-angled at B and ∠A = 300. If BC = 6cm, find (i) AB, (ii) AC.
In the adjoining figure, ΔABC is right-angled at B and ∠A = 450. If AC = 3`sqrt(2)`cm, find (i) BC, (ii) AB.
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
cosB = `(4)/(5)`
In an isosceles triangle ABC, AB = BC = 6 cm and ∠B = 90°. Find the values of cosec C
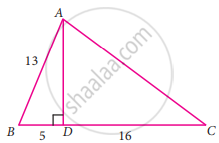
In the given figure, AD is the median on BC from A. If AD = 8 cm and BC = 12 cm, find the value of cos y
In the given figure, AD is the median on BC from A. If AD = 8 cm and BC = 12 cm, find the value of tan x. cot y
If cosec θ = `(29)/(20)`, find the value of: cosec θ - `(1)/("cot" θ)`
From the given figure, find the values of cot B