Advertisements
Advertisements
Question
From the given figure, find the values of cot B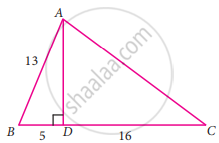
Solution
In the right ΔABD,
AD2 = AB2 – BD2
= 132 – 52
= 169 – 25
= 144
AD = `sqrt(144)`
= 12
In the right ΔADC,
AC2 = AD2 + DC2
= 122 + 162
= 144 + 256
= 400
AC = `sqrt(400)`
= 20
cot B = `"adjacent side"/"opposite side" = "BD"/"AB" = 5/12`
APPEARS IN
RELATED QUESTIONS
If sin θ = ` (a^2 - b^2)/(a^2+b^2)`find all the values of all T-ratios of θ .
Using the formula, tan 2A =`(2 tan A )/(1- tan^2 A)` find the value of tan 600, it being given that tan 300 = `1/sqrt(3)`.
In the adjoining figure, ΔABC is right-angled at B and ∠A = 450. If AC = 3`sqrt(2)`cm, find (i) BC, (ii) AB.
Given: sec A = `( 29 )/(21), "evaluate : sin A" - 1/tan "A"`
Given : 17 cos θ = 15;
Find the value of: tan θ + 2 secθ .
If sin A = cos A, find the value of 2 tan2A - 2 sec2 A + 5.
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
cotA = `(1)/(11)`
If sin θ = `(8)/(17)`, find the other five trigonometric ratios.
In the given figure, PQR is a triangle, in which QS ⊥ PR, QS = 3 cm, PS = 4 cm and QR = 12 cm, find the value of: 4sin2R - `(1)/("tan"^2"P")`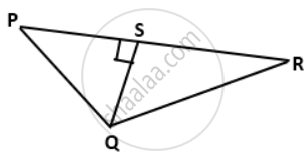
In the given figure, AD is the median on BC from A. If AD = 8 cm and BC = 12 cm, find the value of `(1)/("sin"^2 x) - (1)/("tan"^2 x)`
