Advertisements
Advertisements
Question
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
cotA = `(1)/(11)`
Solution
cotA = `(1)/(11)`
cotA = `(1)/"tanA" ="Base"/"Perpendicular"`
By Pythagoras theorem, we have
(Hypotenuse)2 = (Perpendicular)2 + (Base)2
(Hypotenuse) = `sqrt(("Perpendicular")^2 + ("Base")^2`
= `sqrt((11)^2 + (1)^2`
= `sqrt(121 + 1)`
= `sqrt(122)`
cosA = `"Base"/"Hypotenuse" = (1)/sqrt(122)`
tanA = `"Perpendicular"/"Base"` = 11
secA = `(1)/"cosA" = sqrt(122)`
sinA = `"Perpendicular"/"Hypotenuse" = (11)/sqrt(122)`
cosecA = `(1)/"sinA" = sqrt(122)/(11)`.
APPEARS IN
RELATED QUESTIONS
If `sin A = 9/41` compute cos 𝐴 𝑎𝑛𝑑 tan 𝐴
if `sec A = 5/4` verify that `(3 sin A - 4 sin^3 A)/(4 cos^3 A - 3 cos A) = (3 tan A - tan^3 A)/(1- 3 tan^2 A)`
If A = 30° and B = 60°, verify that cos (A + B) = cos A cos B − sin A sin B
In rectangle ABCD AB = 20cm ∠BAC = 60° BC, calculate side BC and diagonals AC and BD.
If 3tan θ 4 , show that `((4cos theta - sin theta ))/((4 cos theta + sin theta))=4/5`
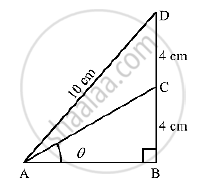
In the adjoining figure, `∠B = 90° , ∠BAC = theta° , BC = CD = 4cm and AD = 10 cm`. find (i) sin theta and (ii) `costheta`
If A = 600 and B = 300, verify that:
(i) sin (A – B) = sin A cos B – cos A sin B
If sin A + cosec A = 2;
Find the value of sin2 A + cosec2 A.
In the given figure, PQR is a triangle, in which QS ⊥ PR, QS = 3 cm, PS = 4 cm and QR = 12 cm, find the value of: cot2P - cosec2P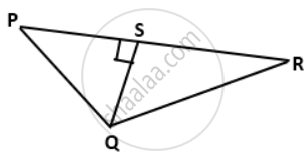
In the given figure, ΔABC is right angled at B.AD divides BC in the ratio 1 : 2. Find
(i) `("tan"∠"BAC")/("tan"∠"BAD")` (ii) `("cot"∠"BAC")/("cot"∠"BAD")`
