Advertisements
Advertisements
Question
If sin A = cos A, find the value of 2 tan2A - 2 sec2 A + 5.
Solution
Consider the figure : 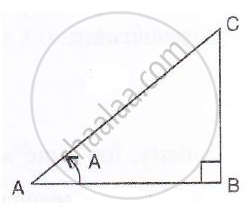
sin A = cos A
tan A = `(1)/(1)`
i.e.`"perpendicular"/"base" = "BC"/"AB" = (1)/(1)`
Therefore if length of perpendicular = x, length of base = x
Since
AB2 + BC2 = AC2 ...[ Using Pythagoras Theorem]
(x)2 + (x)2 = AC2
AC2 = 2x2
∴ AC = `sqrt2x`
Now
sec A = `"AC"/"AB" = sqrt2`
Therefore
2 tan2 A – 2sec2 A + 5
= 2(1)2 –2 (`sqrt2`)2 + 5
= 2 – 4 + 5
= 3
APPEARS IN
RELATED QUESTIONS
If `sin A = 9/41` compute cos 𝐴 𝑎𝑛𝑑 tan 𝐴
if `sin theta = 3/5 " evaluate " (cos theta - 1/(tan theta))/(2 cot theta)`
If cos 2θ = sin 4θ where 2θ, 4θ are acute angles, find the value of θ.
If 3tan θ 4 , show that `((4cos theta - sin theta ))/((4 cos theta + sin theta))=4/5`
Evaluate:
cos450 cos300 + sin450 sin300
If A = 300 , verify that:
(i) sin 2A = `(2 tan A)/(1+tan^2A)`
Given : 17 cos θ = 15;
Find the value of: tan θ + 2 secθ .
If sec A = `sqrt2` , find : `(3cot^2 "A"+ 2 sin^2 "A")/ (tan^2 "A" – cos ^2 "A")`.
In a right-angled triangle PQR, ∠PQR = 90°, QS ⊥ PR and tan R =`(5)/(12)`, find the value of tan ∠SQR
From the given figure, find all the trigonometric ratios of angle B
