Advertisements
Advertisements
Question
In rectangle ABCD, diagonal BD = 26 cm and cotangent of angle ABD = 1.5. Find the area and the perimeter of the rectangle ABCD.
Solution
Consider the diagram:
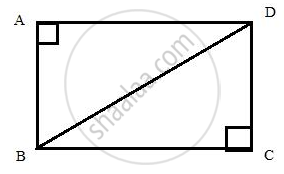
cot ∠ABD = `(15)/(10) = (3)/(2)`
i.e.`"base"/"perpendicular" = "AB"/"BD" = (3)/(2)`
Therefore, if length of base = 3x, length of perpendicular = 2x
Since
AB2 + AD2 = BD2 ...[Using Pythagoras Theorem]
(3x)2 + (2x)2 = BD2
BD2 = 13x2
∴ BD = `sqrt13x`
Now
BD = 26
`sqrt13x` = 26
x = `(26)/sqrt13`
Therefore
AD = 2x
= 2x `(26)/sqrt13`
= `(52)/sqrt13` cm
AB = 3x
= `3 xx (26)/sqrt13`
=`(78)/sqrt13` cm
Now
Area of rectangle ABCD = AB × AD
= `(78)/sqrt13 x (52)/sqrt13`
= 312 cm2
Perim of rectangle ABCD = 2 (AB + AD)
= 2 `((78)/sqrt13 + (52)/sqrt13)`
= `(260)/sqrt13`
= 20`sqrt13` cm
APPEARS IN
RELATED QUESTIONS
If θ = 30° verify `tan 2 theta = (2 tan theta)/(1 - tan^2 theta)`
If cos θ = `3/5` , show that `((sin theta - cot theta ))/(2tan theta)=3/160`
Verify each of the following:
(i)`sin 60^0 cos 30^0-cos 60^0 sin 30^0`
If A = 600 and B = 300, verify that:
cos (A + B) = cos A cos B - sin A sin B
If A and B are acute angles such that tan A =`1/3, tan B = 1/2 and tan (A + B) =` show that `A+B = 45^0`
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
cotA = `(1)/(11)`
In each of the following, one trigonometric ratio is given. Find the values of the other trigonometric.
cose C = `(15)/(11)`
In the given figure, ΔABC is right angled at B.AD divides BC in the ratio 1 : 2. Find
(i) `("tan"∠"BAC")/("tan"∠"BAD")` (ii) `("cot"∠"BAC")/("cot"∠"BAD")`
In the given figure, AC = 13cm, BC = 12 cm and ∠B = 90°. Without using tables, find the values of: sin A cos A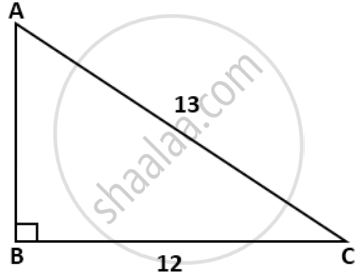
From the given figure, find all the trigonometric ratios of angle B
