Advertisements
Advertisements
Question
It is possible to have a triangle in which each angle is greater than 60°.
Options
True
False
Solution
This statement is False.
Explanation:
If all angles of a triangle are greater than 60°, then their sum will be greater than 180°. But in a triangle sum of all angles is 180°
∴ Triangle is not possible.
APPEARS IN
RELATED QUESTIONS
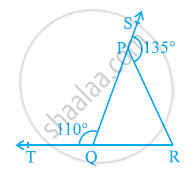
In the given figure, sides QP and RQ of ΔPQR are produced to points S and T respectively. If ∠SPR = 135º and ∠PQT = 110º, find ∠PRQ.
Find the value of the unknowns x and y in the following diagram:

Find the value of the unknown x and y in the following diagram:

In the following triangle, find the value of x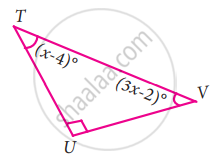
In ∆RST, ∠S is 10° greater than ∠R and ∠T is 5° less than ∠S, find the three angles of the triangle
In the given figure, PQ = PS. The value of x is ______.

In a triangle, one angle is of 90°. Then ______.
- The other two angles are of 45° each
- In remaining two angles, one angle is 90° and other is 45°
- Remaining two angles are complementary
In the given option(s) which is true?
In ΔPQR of the given figure, PQ = PR. Find the measures of ∠Q and ∠R.
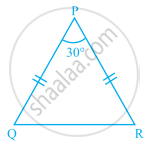
The angles of a triangle are arranged in descending order of their magnitudes. If the difference between two consecutive angles is 10°, find the three angles.
In ∆ABC, DE || BC (see figure). Find the values of x, y and z.

