Advertisements
Advertisements
Question
Find the value of the unknown x and y in the following diagram:

Solution

y = x (Vertically opposite angles)
a = x (Vertically opposite angles)
b = x (Vertically opposite angles)
a + b + y = 180° (Angle sum property)
x + x + x = 180°
3x = 180°
x =
x = 60°
y = x = 60°
APPEARS IN
RELATED QUESTIONS
In the given figure, the side QR of ΔPQR is produced to a point S. If the bisectors of ∠PQR and ∠PRS meet at point T, then prove that ∠QTR = 1/2∠QPR.

In the following triangle, find the value of x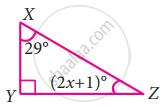
In ∆RST, ∠S is 10° greater than ∠R and ∠T is 5° less than ∠S, find the three angles of the triangle
If ∆MNO ≅ ∆DEF, ∠M = 60° and ∠E = 45° then find the value of ∠O
Can a triangle have two obtuse angles? Give reason for your answer.
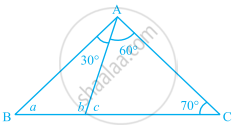
In the given figure, find the values of a, b and c.
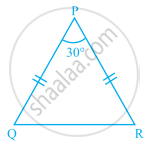
In ΔPQR of the given figure, PQ = PR. Find the measures of ∠Q and ∠R.
Each of the two equal angles of an isosceles triangle is four times the third angle. Find the angles of the triangle.
The angles of a triangle are arranged in descending order of their magnitudes. If the difference between two consecutive angles is 10°, find the three angles.
In the given figure, if ST = SU, then find the values of x and y.

