Advertisements
Advertisements
Question
The angles of a triangle are arranged in descending order of their magnitudes. If the difference between two consecutive angles is 10°, find the three angles.
Solution
Let one of the angles of a triangle be x. If angles are arranged in descending order. Then, angles will be x, (x – 10°) and (x – 20°).
We know that the sum of all angles in a triangle is equal to 180°
So, x + (x – 10°) + (x – 20°) = 180°
⇒ x + x + x – 30° = 180°
⇒ 3x = 180° + 30°
⇒ 3x = 210°
⇒ x = `210^circ/3` = 70°
Hence, angles will be 70°, 70° – 10° and 70° – 20° i.e., 70°, 60° and 50°.
APPEARS IN
RELATED QUESTIONS
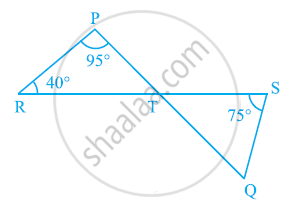
In the given figure, if lines PQ and RS intersect at point T, such that ∠PRT = 40º, ∠RPT = 95º and ∠TSQ = 75º, find ∠SQT.
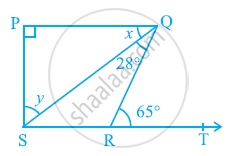
In the given figure, if PQ ⊥ PS, PQ || SR, ∠SQR = 28º and ∠QRT = 65º, then find the values of x and y.
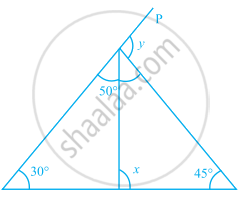
Find the value of the unknown x and y in the following diagram:
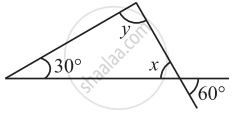
Find the value of the unknown x and y in the following diagram:

If ∆MNO ≅ ∆DEF, ∠M = 60° and ∠E = 45° then find the value of ∠O
If one of the angles of a triangle is 130°, then the angle between the bisectors of the other two angles can be ______.
How many triangles can be drawn having its angles as 53°, 64° and 63°? Give reason for your answer.
Prove that a triangle must have atleast two acute angles.
In the given figure, ∠BAC = 90°, AD ⊥ BC and ∠BAD = 50°, then ∠ACD is ______.

Find the values of x and y in the given figure.