Advertisements
Advertisements
Question
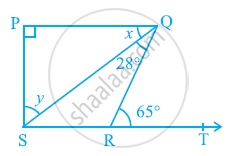
In the given figure, if PQ ⊥ PS, PQ || SR, ∠SQR = 28º and ∠QRT = 65º, then find the values of x and y.
Solution
It is given that PQ || SR and QR is a transversal line.
∠PQR = ∠QRT (Alternate interior angles)
x + 28º = 65º
x = 65º − 28º
x = 37º
By using the angle sum property for ΔSPQ, we obtain
∠SPQ + x + y = 180º
90º + 37º + y = 180º
y = 180º − 127º
y = 53º
∴x = 37º and y = 53º
APPEARS IN
RELATED QUESTIONS
In the given figure, the side QR of ΔPQR is produced to a point S. If the bisectors of ∠PQR and ∠PRS meet at point T, then prove that ∠QTR = 1/2∠QPR.

Find the value of the unknown x in the following diagram:

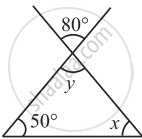
Find the value of the unknown x and y in the following diagram:
In the following triangle, find the value of x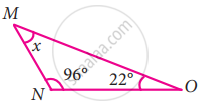
In ∆ABC, if ∠B is 3 times ∠A and ∠C is 2 times ∠A, then find the angle
If ∆MNO ≅ ∆DEF, ∠M = 60° and ∠E = 45° then find the value of ∠O
Angles of a triangle are in the ratio 2 : 4 : 3. The smallest angle of the triangle is ______.
The angles of a triangle are in the ratio 2 : 3 : 4. Find the angles of the triangle.
In triangle XYZ, the measure of angle X is 30° greater than the measure of angle Y and angle Z is a right angle. Find the measure of ∠Y.
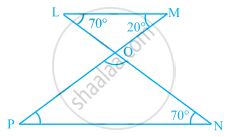
In the given figure, find the measures of ∠PON and ∠NPO.