Advertisements
Advertisements
Question
Point P (2, -7) is the center of a circle with radius 13 unit, PT is perpendicular to chord AB and T = (-2, -4); calculate the length of: AT
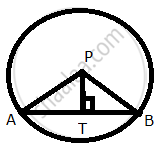
Solution
Given, radius = 13 units
PA = PB = 13 units
Using distance formula,
PT = `sqrt((-2 -2)^2 + (-4 + 7)^2)`
= `sqrt(16 + 9)`
= `sqrt(25)`
= 5
Using Pythagoras theorem in Δ PAT,
AT2 = PA2 - PT2
AT2 = 169 - 25
AT2 = 144
AT = 12 units.
APPEARS IN
RELATED QUESTIONS
Name the type of quadrilateral formed, if any, by the following points, and give reasons for your answer:
(- 1, - 2), (1, 0), (- 1, 2), (- 3, 0)
Find the distance between the following pair of points:
(-6, 7) and (-1, -5)
Find the distance between the points
A(-6,-4) and B(9,-12)
Find the distance of the following points from the origin:
(iii) C (-4,-6)
Show that the ▢PQRS formed by P(2, 1), Q(–1, 3), R(–5, –3) and S(–2, –5) is a rectangle.
Find the distance of the following point from the origin :
(8 , 15)
Find the distance of the following point from the origin :
(13 , 0)
Find the distance of a point (7 , 5) from another point on the x - axis whose abscissa is -5.
Find the coordinate of O , the centre of a circle passing through P (3 , 0), Q (2 , `sqrt 5`) and R (`-2 sqrt 2` , -1). Also find its radius.
A(-2, -3), B(-1, 0) and C(7, -6) are the vertices of a triangle. Find the circumcentre and the circumradius of the triangle.
Prove that the points (5 , 3) , (1 , 2), (2 , -2) and (6 ,-1) are the vertices of a square.
Prove that the points (0 , 2) , (1 , 1) , (4 , 4) and (3 , 5) are the vertices of a rectangle.
Prove that the points (0 , -4) , (6 , 2) , (3 , 5) and (-3 , -1) are the vertices of a rectangle.
Find the distance between the following pairs of point:
`(sqrt(3)+1,1)` and `(0, sqrt(3))`
Calculate the distance between the points P (2, 2) and Q (5, 4) correct to three significant figures.
By using the distance formula prove that each of the following sets of points are the vertices of a right angled triangle.
(i) (6, 2), (3, -1) and (- 2, 4)
(ii) (-2, 2), (8, -2) and (-4, -3).
Show that the points (a, a), (-a, -a) and `(-asqrt(3), asqrt(3))` are the vertices of an equilateral triangle.
Find distance between point Q(3, – 7) and point R(3, 3)
Solution: Suppose Q(x1, y1) and point R(x2, y2)
x1 = 3, y1 = – 7 and x2 = 3, y2 = 3
Using distance formula,
d(Q, R) = `sqrt(square)`
∴ d(Q, R) = `sqrt(square - 100)`
∴ d(Q, R) = `sqrt(square)`
∴ d(Q, R) = `square`
The equation of the perpendicular bisector of line segment joining points A(4,5) and B(-2,3) is ______.
Show that points A(–1, –1), B(0, 1), C(1, 3) are collinear.
