Advertisements
Advertisements
Question
Prove that in a right-angle triangle, the square of the hypotenuse is equal to the sum of squares of the other two sides.
Solution
Given: A right triangle ABC in which ∠B = 90°
To Prove: (Hypotenuse)2 = (Base)2 + (perpendicular)2
i.e AC2 = AB2 + BC2
Construction: From B, Draw BD ⊥ AC

In ΔABC and ΔADB
∠BAC = ∠DAB [Common]
∠ABC = ∠ADB [each 90°]
∴ ΔABC ∼ ΔADB [By AA similarity]
`=> "AB"/"AC" = "AD"/"AB"`
`=> AB2 - AD x AC ...(i)
Similarity, ΔABC ∼ ΔBDC
`=> "BC"/"DC" = "AC"/"BC"`
`=> "BC"^2 - "AC" xx "DC"` ...(ii)
On adding (i) and (iii) we get
AB2 + BC2 = AD x AC + AC x DC
`=>` AB2 + BC2 = AC(AD + DC)
`=>` AB2 + BC2 = AC x AC
`=>` AC2 = AB2 + BC2
Notes
Δ
∠
APPEARS IN
RELATED QUESTIONS
In two similar triangles ABC and PQR, if their corresponding altitudes AD and PS are in the ratio 4 : 9, find the ratio of the areas of ∆ABC and ∆PQR
If ∆ABC is similar to ∆DEF such that ∆DEF = 64 cm2 , DE = 5.1 cm and area of ∆ABC = 9 cm2 . Determine the area of AB
Let Δ ABC ~ Δ DEF and their areas be, respectively, 64 cm2 and 121 cm2. If EF = 15.4 cm, find BC
In figure below ΔACB ~ ΔAPQ. If BC = 10 cm, PQ = 5 cm, BA = 6.5 cm and AP = 2.8 cm,
find CA and AQ. Also, find the area (ΔACB): area (ΔAPQ)

The areas of two similar triangles are 81 cm2 and 49 cm2 respectively. Find the ratio of their corresponding heights. What is the ratio of their corresponding medians?
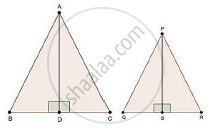
The areas of two similar triangles are 121 cm2 and 64 cm2 respectively. If the median of the first triangle is 12.1 cm, find the corresponding median of the other.
In the given figure 1.66, seg PQ || seg DE, A(∆PQF) = 20 units, PF = 2 DP, then Find A(◻DPQE) by completing the following activity.

In ΔLMN, ∠L = 50° and ∠N = 60°, If ΔLMN ~ ΔPQR, then find ∠Q.
O is a point on side PQ of a APQR such that PO = QO = RO, then ______.
If ΔABC ∼ ΔPQR and `(A(ΔABC))/(A(ΔPQR)) = 16/25`, then find AB : PQ.
