English Medium
Academic Year: 2018-2019
Date: March 2019
Duration: 3h
Advertisements
1. All questions are compulsory.
2. This question paper consists of 30 questions divided into four Section- A, B,C and D.
3. Section A contains 6 questions of 1 marks each.
Section B contains 6 questions of 2 marks each.
Section C contains 10 questions of 3 marks each.
Section D contains 8 questions of 4 marks each.
Find the coordinates of a point A, where AB is the diameter of circle whose centre is (2, -3) and B is (1, 4).
Chapter: [0.031] Lines (In Two-dimensions)
For what values of k, the roots of the equation x2 + 4x +k = 0 are real?
Chapter: [0.023] Quadratic Equations
Find the value of k for which the roots of the equation 3x2 -10x +k = 0 are reciprocal of each other.
Chapter: [0.023] Quadratic Equations
Find A if tan 2A = cot (A-24°).
Chapter: [0.051] Introduction to Trigonometry [0.052000000000000005] Trigonometric Identities
Find the value of ( sin2 33° + sin2 57°).
Chapter: [0.051] Introduction to Trigonometry [0.052000000000000005] Trigonometric Identities
How many two digits numbers are divisible by 3?
Chapter: [0.011000000000000001] Real Numbers
In fig.DE || BC ,AD = 1 cm and BD = 2 cm. what is the ratio of the ar(ΔABC) to the ar (ΔADE)?

Chapter: [0.040999999999999995] Triangles [0.040999999999999995] Triangles [0.040999999999999995] Triangles
Find a rational number between `sqrt(2) " and sqrt(3) `.
Chapter: [0.011000000000000001] Real Numbers
Find the HCF of 1260 and 7344 using Euclid's algorithm.
Chapter: [0.011000000000000001] Real Numbers
Show that every positive odd integer is of the form (4q +1) or (4q+3), where q is some integer.
Chapter: [0.021] Polynomials
Which term of the AP 3, 15, 27, 39, ...... will be 120 more than its 21st term?
Chapter: [0.024] Arithmetic Progressions
If Sn.., the sum of first n terms of an AP is given by Sn =3n2- 4n, find the nth term.
Chapter: [0.024] Arithmetic Progressions
Find the ratio In which is the segment joining the points (1, - 3} and (4, 5) ls divided by x-axis? Also, find the coordinates of this point on the x-axis.
Chapter: [0.031] Lines (In Two-dimensions)
Advertisements
A game consists of tossing a coin 3 times and noting the outcome each time. If getting the result in the tosses is a success, find the probability of losing the game.
Chapter: [0.07200000000000001] Probability [0.07200000000000001] Probability
A die is thrown once. Find the probability of getting a number which (i) is a prime number (ii) lies between 2 and 6.
Chapter: [0.07200000000000001] Probability [0.07200000000000001] Probability
Find c if the system of equations cx+3y+(3 - c ) = 0, 12x + cy - c = 0 has infinitely many solutions?
Chapter: [0.023] Quadratic Equations
Prove that is `sqrt2` irrational number.
Chapter: [0.011000000000000001] Real Numbers
Find the value of k such that the polynomial x2-(k +6)x+ 2(2k - 1) has some of its zeros equal to half of their product.
Chapter: [0.021] Polynomials
A father's age is three times the sum of the ages of his two children. After 5 years his age will be two times the sum of their ages. Find the present age of the father.
Chapter: [0.07200000000000001] Probability [0.07200000000000001] Probability
A fraction becomes `1/3` when 2 is subtracted from the numerator and it becomes `1/2` when 1 is subtracted from the denominator. Find the fraction.
Chapter: [0.022000000000000002] Pair of Linear Equations in Two Variables
Find the point on the y-axis which is equidistant from the points (S, - 2) and (- 3, 2).
Chapter: [0.031] Lines (In Two-dimensions) [0.031] Lines (In Two-dimensions)
The line segment joining the points A(2, 1) and B (5, - 8) is trisected at the points P and Q such that P is nearer to A. If P also lies on the line given by 2x - y + k= 0 find the value of k.
Chapter: [0.031] Lines (In Two-dimensions) [0.031] Lines (In Two-dimensions)
Prove that :(sinθ+cosecθ)2+(cosθ+ secθ)2 = 7 + tan2 θ+cot2 θ.
Chapter: [0.051] Introduction to Trigonometry [0.052000000000000005] Trigonometric Identities
Prove that: (1+cot A - cosecA)(1 + tan A+ secA) =2.
Chapter: [0.051] Introduction to Trigonometry [0.052000000000000005] Trigonometric Identities
ln Figure, PQ is a chord of length 8 cm of a circle of radius 5 cm and centre O. The tangents at P and Q intersect at point T. find the length of TP.
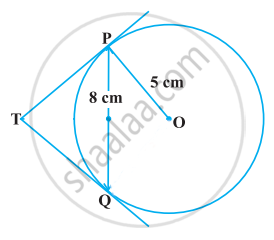
Chapter: [0.042] Circles
In the following Figure ∠ACB= 90° and CD ⊥ AB, prove that CD2 = BD × AD

Chapter: [0.040999999999999995] Triangles
Advertisements
If P and Q are the points on side CA and CB respectively of ΔABC, right angled at C, prove that (AQ2 + BP2) = (AB2 + PQ2)
Chapter: [0.040999999999999995] Triangles
Find the area of the shaded region in the figure If ABCD is a rectangle with sides 8 cm and 6 cm and O is the centre of the circle. (Take π= 3.14)

Chapter: [0.042] Circles
Water in canal, 6 m wide and 1.5 m deep, is flowing with a speed of 10 km/h. how much area will it irrigate in 30 minutes, if 8 cm of standing water is needed?
Chapter: [0.062] Surface Areas and Volumes
Find the mode of the following frequency distribution.
| Class | 0-10 | 10-20 | 20-30 | 30-40 | 40-50 | 50-60 | 60-70 |
| Frequency | 8 | 10 | 10 | 16 | 12 | 6 | 7 |
Chapter: [0.071] Statistics
Two water taps together can fill a tank in `1 7/8` hours. The tap with a longer diameter takes 2 hours less than the tap with a smaller one to fill the tank separately. Find the time in which each tap can fill the tank separately.
Chapter: [0.023] Quadratic Equations
A boat goes 30 km upstream and 44 km downstream in 10 hours. In 13 hours, it can go 40 km upstream and 55 km downstream. Determine the speed of the stream and that of the boat in still water.
Chapter: [0.023] Quadratic Equations
If the sum of the first four terms of an AP is 40 and that of the first 14 terms is 280. Find the sum of its first n terms.
Chapter: [0.024] Arithmetic Progressions
Prove that `(sin "A" - cos "A" + 1)/(sin "A" + cos "A" - 1) = 1/(sec "A" - tan "A")`
Chapter: [0.051] Introduction to Trigonometry [0.051] Introduction to Trigonometry
A man in a boat rowing away from a lighthouse 100 m high takes 2 minutes to change the angle of elevation of the top of the lighthouse from 60° to 30°. Find the speed of the boat in metres per minute [Use `sqrt3` = 1.732]
Chapter: [0.051] Introduction to Trigonometry [0.051] Introduction to Trigonometry
Two poles of equal heights are standing opposite each other an either side of the road, which is 80 m wide. From a point between them on the road, the angles of elevation of the top of the poles are 60° and 30º, respectively. Find the height of poles and the distance of the point from the poles.
Chapter: [0.053] Some Applications of Trigonometry
Construct a ΔABC in which CA = 6 cm, AB = 5 cm and ∠BAC = 45°. Then construct a triangle whose sides are `3/5` of the corresponding sides of ΔABC.
Chapter: [0.040999999999999995] Triangles [0.040999999999999995] Triangles [0.040999999999999995] Triangles
A bucket open at the top is in the form of a frustum of a cone with a capacity of 12308.8 cm3 . the radii of the top and bottom of the circular ends of the bucket are 20 cm and 12 cm respectively. Find the height of the bucket and also the area of the metal sheet used in making it.
Chapter: [0.062] Surface Areas and Volumes
Prove that in a right-angle triangle, the square of the hypotenuse is equal to the sum of squares of the other two sides.
Chapter: [0.040999999999999995] Triangles
If the median of the following frequency distribution is 32.5, find the values of `f_1 and f_2`.
| Class | 0 – 10 | 10 – 20 | 20 – 30 | 30 -40 | 40 – 50 | 50 – 60 | 60 – 70 | Total |
| Frequency | `f_1` |
5 |
9 | 12 | `f_2` | 3 | 2 | 40 |
Chapter: [0.071] Statistics
The marks obtained by 100 students of a class in an examination are given below.
| Marks | No. of students |
| 0-5 | 2 |
| 5-10 | 5 |
| 10-15 | 6 |
| 15-20 | 8 |
| 20-25 | 10 |
| 25-30 | 25 |
| 30-35 | 20 |
| 35-40 | 18 |
| 40-45 | 4 |
| 45-50 | 2 |
Draw 'a less than' type cumulative frequency curves (orgive). Hence find median
Chapter: [0.071] Statistics
Other Solutions
Submit Question Paper
Help us maintain new question papers on Shaalaa.com, so we can continue to help studentsonly jpg, png and pdf files
CBSE previous year question papers Class 10 Mathematics with solutions 2018 - 2019
Previous year Question paper for CBSE Class 10 Maths-2019 is solved by experts. Solved question papers gives you the chance to check yourself after your mock test.
By referring the question paper Solutions for Mathematics, you can scale your preparation level and work on your weak areas. It will also help the candidates in developing the time-management skills. Practice makes perfect, and there is no better way to practice than to attempt previous year question paper solutions of CBSE Class 10.
How CBSE Class 10 Question Paper solutions Help Students ?
• Question paper solutions for Mathematics will helps students to prepare for exam.
• Question paper with answer will boost students confidence in exam time and also give you an idea About the important questions and topics to be prepared for the board exam.
• For finding solution of question papers no need to refer so multiple sources like textbook or guides.
