Advertisements
Advertisements
Question
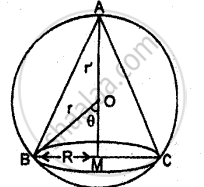
Show that the altitude of the right circular cone of maximum volume that can be inscribed in a sphere of radius r is `(4r)/3.`
Advertisements
Solution
Let the radius of the sphere = r
Radius of cone = R
Height of the cone = AM
= OA + OM
= r + r cos θ
= r(1 + cosθ)
where ∠BOM = θ
BC = diameter of the base of the cone
∴ Radius of cone = r sin θ
Volume of cone V = `1/3 pi (r sin theta)^2 xx r (1 + cos theta)` ....`[because "volume of cone" = 1/3 pir^2 h]`
`= 1/3 pir^3 sin^2 theta (1 + cos theta)`
On differentiating,
`(dV)/(d theta) = 1/3 pir^3 [2 sin theta cos theta (1 + cos theta) + sin^2 theta (- sin theta)]`
`= 1/3 pir^3 [2 sin theta cos theta (1 + cos theta) - sin^3 theta]`
`= 1/3 pir^3 sin theta [2 cos theta (1 + cos theta) - sin^2 theta]`
`= 1/3 pir^3 sin theta [2 cos theta + 2 cos^2 theta - 1+ cos^2 theta]`
`= 1/3 pir^3 sin theta [3 cos^2 theta + 2 cos theta - 1]`
`= 1/3 pir^3 sin theta (cos theta + 1)(3 cos theta - 1)`
For maximum and minimum, `(dV)/(d theta) = 0`
⇒ cos θ ≠ - 1
⇒ θ ≠ π
∴ (3 cos θ - 1) = 0
⇒ `cos theta = 1/3`
In the interval `(0, pi/2)` cos θ is decreasing, cos θ increases as θ decreases and decreases as θ increases.
⇒ at cos θ = `1/3`
The sign of `(dV)/(d theta)` changes from positive to negative as θ passes through this point.
Hence V is highest at this point.
Height of the cone = `r (1 + cos theta) = r(1 + 1/3)`
`= r xx 4/3`
= `(4r)/3`
APPEARS IN
RELATED QUESTIONS
An open box is to be made out of a piece of a square card board of sides 18 cms by cutting off equal squares from the comers and turning up the sides. Find the maximum volume of the box.
If the sum of lengths of hypotenuse and a side of a right angled triangle is given, show that area of triangle is maximum, when the angle between them is π/3.
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
f(x) = x3 − 6x2 + 9x + 15
Find the absolute maximum value and the absolute minimum value of the following function in the given interval:
`f(x) = 4x - 1/x x^2, x in [-2 ,9/2]`
Find both the maximum value and the minimum value of 3x4 − 8x3 + 12x2 − 48x + 25 on the interval [0, 3].
It is given that at x = 1, the function x4− 62x2 + ax + 9 attains its maximum value, on the interval [0, 2]. Find the value of a.
Find two positive numbers x and y such that x + y = 60 and xy3 is maximum.
Find two positive numbers whose sum is 16 and the sum of whose cubes is minimum.
A rectangular sheet of tin 45 cm by 24 cm is to be made into a box without top, by cutting off square from each corner and folding up the flaps. What should be the side of the square to be cut off so that the volume of the box is the maximum possible?
A window is in the form of a rectangle surmounted by a semicircular opening. The total perimeter of the window is 10 m. Find the dimensions of the window to admit maximum light through the whole opening
A given quantity of metal is to be cast into a half cylinder with a rectangular base and semicircular ends. Show that in order that the total surface area may be minimum the ratio of the length of the cylinder to the diameter of its semi-circular ends is \[\pi : (\pi + 2)\].
Find the point on the straight line 2x+3y = 6, which is closest to the origin.
Find the maximum and minimum of the following functions : f(x) = x3 – 9x2 + 24x
Find the maximum and minimum of the following functions : f(x) = `x^2 + (16)/x^2`
Choose the correct option from the given alternatives :
If f(x) = `(x^2 - 1)/(x^2 + 1)`, for every real x, then the minimum value of f is ______.
Solve the following : Show that of all rectangles inscribed in a given circle, the square has the maximum area.
Solve the following:
Find the maximum and minimum values of the function f(x) = cos2x + sinx.
If f(x) = px5 + qx4 + 5x3 - 10 has local maximum and minimum at x = 1 and x = 3 respectively then (p, q) = ______.
The maximum volume of a right circular cylinder if the sum of its radius and height is 6 m is ______.
Show that the function f(x) = 4x3 – 18x2 + 27x – 7 has neither maxima nor minima.
If the sum of the surface areas of cube and a sphere is constant, what is the ratio of an edge of the cube to the diameter of the sphere, when the sum of their volumes is minimum?
The smallest value of the polynomial x3 – 18x2 + 96x in [0, 9] is ______.
The maximum value of `(1/x)^x` is ______.
Find both the maximum and minimum values respectively of 3x4 - 8x3 + 12x2 - 48x + 1 on the interval [1, 4].
The distance of that point on y = x4 + 3x2 + 2x which is nearest to the line y = 2x - 1 is ____________.
For all real values of `x`, the minimum value of `(1 - x + x^2)/(1 + x + x^2)`
A wire of length 22 m is to be cut into two pieces. One of the pieces is to be made into a square and the other into an equilateral triangle. Then, the length of the side of the equilateral triangle, so that the combined area of the square and the equilateral triangle is minimum, is ______.
The range of a ∈ R for which the function f(x) = `(4a - 3)(x + log_e5) + 2(a - 7)cot(x/2)sin^2(x/2), x ≠ 2nπ, n∈N` has critical points, is ______.
Let x and y be real numbers satisfying the equation x2 – 4x + y2 + 3 = 0. If the maximum and minimum values of x2 + y2 are a and b respectively. Then the numerical value of a – b is ______.
The set of values of p for which the points of extremum of the function f(x) = x3 – 3px2 + 3(p2 – 1)x + 1 lie in the interval (–2, 4), is ______.
The minimum value of 2sinx + 2cosx is ______.
The minimum value of the function f(x) = xlogx is ______.
A straight line is drawn through the point P(3, 4) meeting the positive direction of coordinate axes at the points A and B. If O is the origin, then minimum area of ΔOAB is equal to ______.
Check whether the function f : R `rightarrow` R defined by f(x) = x3 + x, has any critical point/s or not ? If yes, then find the point/s.
If Mr. Rane order x chairs at the price p = (2x2 - 12x - 192) per chair. How many chairs should he order so that the cost of deal is minimum?
Solution: Let Mr. Rane order x chairs.
Then the total price of x chairs = p·x = (2x2 - 12x- 192)x
= 2x3 - 12x2 - 192x
Let f(x) = 2x3 - 12x2 - 192x
∴ f'(x) = `square` and f''(x) = `square`
f'(x ) = 0 gives x = `square` and f''(8) = `square` > 0
∴ f is minimum when x = 8
Hence, Mr. Rane should order 8 chairs for minimum cost of deal.
If x + y = 8, then the maximum value of x2y is ______.
Divide the number 100 into two parts so that the sum of their squares is minimum.
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
f(x) `= x sqrt(1 - x), 0 < x < 1`
The shortest distance between the line y - x = 1and the curve x = y2 is
