Advertisements
Advertisements
Question
Solve the following quadratic equation by factorization method
`sqrt("a"("a" - 7)) = 3sqrt(2)`
Solution
`sqrt("a"("a" - 7)) = 3sqrt(2)`
Squaring on both sides
a(a – 7) = `(3sqrt(2))^2`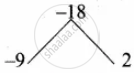
a2 – 7a = 18
a2 – 7a – 18 = 0
(a – 9) (a + 2) = 0
a – 9 = 0 or a + 2 = 0
The roots are – 2 and 9
APPEARS IN
RELATED QUESTIONS
Find the sum and product of the roots for the following quadratic equation
`3 + 1/"a" = 10/"a"^2`
Find the sum and product of the roots for the following quadratic equation
3y2 – y – 4 = 0
Solve the following quadratic equation by formula method
2x2 – 5x + 2 = 0
Solve the following quadratic equation by formula method
3y2 – 20y – 23 = 0
From a group of 2x2 black bees, square root of half of the group went to a tree. Again eight-ninth of the bees went to the same tree. The remaining two got caught up in a fragrant lotus. How many bees were there in total?
There is a square field whose side is 10 m. A square flower bed is prepared in its centre leaving a gravel path all round the flower bed. The total cost of laying the flower bed and gravelling the path at ₹ 3 and ₹ 4 per square metre respectively is ₹ 364. Find the width of the gravel path
Determine the nature of the roots for the following quadratic equation
`sqrt(2)"t"^2 - 3"t" + 3sqrt(2)` = 0
If the roots of the equation (c2 – ab)x2 – 2(a2 – bc)x + b2 – ac = 0 are real and equal prove that either a = 0 (or) a3 + b3 + c3 = 3abc
Write the following expression in terms of α + β and αβ
`(alpha + 3)/beta + (beta + 3)/alpha`
The roots of the equation x2 + 6x – 4 = 0 are α, β. Find the quadratic equation whose roots are α2β and β2α
