Advertisements
Advertisements
Question
Suppose the smaller pulley of the previous problem has its radius 5⋅0 cm and moment of inertia 0⋅10 kg-m2. Find the tension in the part of the string joining the pulleys.
Solution
Given
m = 2 kg, I1 = 0.10 kg-m2
r1 = 5 cm = 0.05 m
I2 = 2.20 kg-m2
r2 = 10 cm = 0.1 m
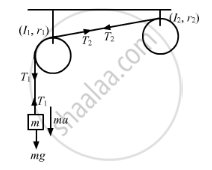
From the free body diagram, we have
\[mg - T_1 = ma....(1)\]
\[\left( T_1 - T_2 \right) r_1 = I_1 \alpha......(2)\]
\[ T_2 r_2 = I_2 \alpha .......(3)\]
Substituting the value of T2 in the equation (2), we get
\[\Rightarrow \left( T_1 - I_2 \frac{\alpha}{r_2} \right) r_1 = I_1 \alpha\]
\[ \Rightarrow T_1 - I_2 \frac{a}{r_2^2} = I_1 \frac{a}{r_1^2}\]
\[ \Rightarrow T_1 = \left\{ \left( \frac{I_1}{r_1^2} \right) + \left( \frac{I_2}{r_2^2} \right) \right\}a\]
Substituting the value of T1 in the equation (1), we get
\[mg - \left\{ \left( \frac{I_1}{r_1^2} \right) + \left( \frac{I_2}{r_2^2} \right) \right\}a = ma\]
\[\Rightarrow \frac{mg}{\left\{ \left( \frac{I_1}{r_1^2} \right) + \left( \frac{I_2}{r_2^2} \right) \right\} + m} = a\]
\[ \Rightarrow a = \frac{2 \times 9 . 8}{\frac{0 . 1}{0 . 0025} + \frac{0 . 2}{0 . 01} + 2}\]
\[= 0 . 316 m/s^2 \]
\[ \Rightarrow T_2 = I_2 \frac{a}{r_2^2}\]
\[ = \frac{0 . 20 \times 0 . 316}{0 . 01} = 6 . 32 N\]
APPEARS IN
RELATED QUESTIONS
Find the moment of inertia of a sphere about a tangent to the sphere, given the moment of inertia of the sphere about any of its diameters to be 2MR2/5, where M is the mass of the sphere and R is the radius of the sphere.
Given the moment of inertia of a disc of mass M and radius R about any of its diameters to be MR2/4, find its moment of inertia about an axis normal to the disc and passing through a point on its edge
Torques of equal magnitude are applied to a hollow cylinder and a solid sphere, both having the same mass and radius. The cylinder is free to rotate about its standard axis of symmetry, and the sphere is free to rotate about an axis passing through its centre. Which of the two will acquire a greater angular speed after a given time?
Show that the child’s new kinetic energy of rotation is more than the initial kinetic energy of rotation. How do you account for this increase in kinetic energy?
A rope of negligible mass is wound round a hollow cylinder of mass 3 kg and radius 40 cm. What is the angular acceleration of the cylinder if the rope is pulled with a force of 30 N? What is the linear acceleration of the rope? Assume that there is no slipping.
A hoop of radius 2 m weighs 100 kg. It rolls along a horizontal floor so that its centre of mass has a speed of 20 cm/s. How much work has to be done to stop it?
The moment of inertia of a uniform semicircular wire of mass M and radius r about a line perpendicular to the plane of the wire through the centre is ___________ .
A body having its centre of mass at the origin has three of its particles at (a,0,0), (0,a,0), (0,0,a). The moments of inertia of the body about the X and Y axes are 0⋅20 kg-m2 each. The moment of inertia about the Z-axis
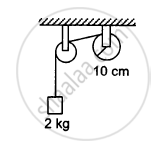
A string is wrapped on a wheel of moment of inertia 0⋅20 kg-m2 and radius 10 cm and goes through a light pulley to support a block of mass 2⋅0 kg as shown in the following figure. Find the acceleration of the block.
The descending pulley shown in the following figure has a radius 20 cm and moment of inertia 0⋅20 kg-m2. The fixed pulley is light and the horizontal plane frictionless. Find the acceleration of the block if its mass is 1⋅0 kg.
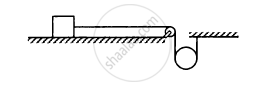
Solve the previous problem if the friction coefficient between the 2⋅0 kg block and the plane below it is 0⋅5 and the plane below the 4⋅0 kg block is frictionless.
A uniform metre stick of mass 200 g is suspended from the ceiling thorough two vertical strings of equal lengths fixed at the ends. A small object of mass 20 g is placed on the stick at a distance of 70 cm from the left end. Find the tensions in the two strings.
Four bodies of masses 2 kg, 3 kg, 4 kg and 5 kg are placed at points A, B, C, and D respectively of a square ABCD of side 1 metre. The radius of gyration of the system about an axis passing through A and perpendicular to plane is
A wheel of mass 15 kg has a moment of inertia of 200 kg-m2 about its own axis, the radius of gyration will be:
From a circular ring of mass, ‘M’ and radius ‘R’ an arc corresponding to a 90° sector is removed. The moment of inertia of the remaining part of the ring about an axis passing through the centre of the ring and perpendicular to the plane of the ring is ‘K’ times ‘MR2’. Then the value of ‘K’ is ______.
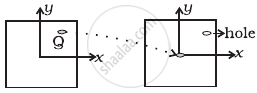
A uniform square plate has a small piece Q of an irregular shape removed and glued to the centre of the plate leaving a hole behind (Figure). The moment of inertia about the z-axis is then ______.
Moment of inertia (M.I.) of four bodies, having same mass and radius, are reported as :
I1 = M.I. of thin circular ring about its diameter,
I2 = M.I. of circular disc about an axis perpendicular to disc and going through the centre,
I3 = M.I. of solid cylinder about its axis and
I4 = M.I. of solid sphere about its diameter.
Then -
Consider a badminton racket with length scales as shown in the figure.
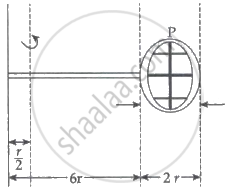
If the mass of the linear and circular portions of the badminton racket is the same (M) and the mass of the threads is negligible, the moment of inertia of the racket about an axis perpendicular to the handle and in the plane of the ring at, `r/2` distance from the ends A of the handle will be ______ Mr2.
