Advertisements
Advertisements
Question
The perpendicular PS on the base QR of a ∆PQR intersects QR at S, such that QS = 3 SR. Prove that 2PQ2 = 2PR2 + QR2
Solution
Given QS = 3SR
QR = QS + SR
= 3SR + SR = 4SR
SR = `1/4` QR ...(1)
QS = 3SR
SR = `"QS"/3` ...(2)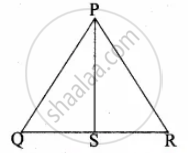
From (1) and (2) we get
`1/4 "QR" = "QS"/3`
∴ QS = `3/4` QR ...(3)
In the right ∆PQS,
PQ2 = PS2 + QS2 ...(4)
Similarly in ∆PSR
PR2 = PS2 + SR2 ...(5)
Subtract (4) and (5)
PQ2 – PR2 = PS2 + QS2 – PS2 – SR2
= QS2 – SR2
PQ2 – PR2 = `[3/4 "QR"]^2 - ["QR"/4]^2`
From (3) and (1)
= `(9"QR"^2)/16 - "QR"^2/16`
= `(8"QR"^2)/16`
PQ2 – PR2 = `1/2 "QR"^2`
2PQ2 – 2PR2 = QR2
2PQ2 = 2PR2 + QR2
Hence the proved.
APPEARS IN
RELATED QUESTIONS
The diagonal of a rectangular field is 16 metres more than the shorter side. If the longer side is 14 metres more than the shorter side, then find the lengths of the sides of the field.
In Figure ABD is a triangle right angled at A and AC ⊥ BD. Show that AC2 = BC × DC

In Figure, ABD is a triangle right angled at A and AC ⊥ BD. Show that AD2 = BD × CD

Two poles of heights 6 m and 11 m stand on a plane ground. If the distance between the feet of the poles is 12 m, find the distance between their tops.
Find the value of (sin2 33 + sin2 57°)
The sides of the triangle are given below. Find out which one is the right-angled triangle?
1.5, 1.6, 1.7
The perimeters of two similar triangles ABC and PQR are 60 cm and 36 cm respectively. If PQ = 9 cm, then AB equals ______.
Prove that the area of the equilateral triangle drawn on the hypotenuse of a right angled triangle is equal to the sum of the areas of the equilateral triangles drawn on the other two sides of the triangle.
In a triangle, sum of squares of two sides is equal to the square of the third side.
Jayanti takes shortest route to her home by walking diagonally across a rectangular park. The park measures 60 metres × 80 metres. How much shorter is the route across the park than the route around its edges?
