Advertisements
Advertisements
Question
The probability distribution of a random variable X is given below:
| x | 0 | 1 | 2 | 3 |
| P(X) | k |
\[\frac{k}{2}\]
|
\[\frac{k}{4}\]
|
\[\frac{k}{8}\]
|
Determine P(X ≤ 2) and P(X > 2) .
Solution
We have,
The probability distribution of a random variable X is given below:
| x | 0 | 1 | 2 | 3 |
| P(X) | k |
\[\frac{k}{2}\]
|
\[\frac{k}{4}\]
|
\[\frac{k}{8}\]
|
\[ \text{ As } , P\left( X \leq 2 \right) = 1 - P\left( X = 3 \right)\]
\[ = 1 - \frac{k}{8}\]
\[ = 1 - \frac{8}{15 \times 8}\]
\[ = 1 - \frac{1}{15}\]
\[ = \frac{14}{15}\]
\[\text{ Also} , P\left( X > 2 \right) = P\left( X = 3 \right)\]
\[ = \frac{8}{15 \times 8}\]
\[ = \frac{1}{15}\]
APPEARS IN
RELATED QUESTIONS
A random variable X has the following probability distribution:
then E(X)=....................
Find the probability distribution of number of heads in two tosses of a coin.
From a lot of 30 bulbs which include 6 defectives, a sample of 4 bulbs is drawn at random with replacement. Find the probability distribution of the number of defective bulbs.
Suppose that two cards are drawn at random from a deck of cards. Let X be the number of aces obtained. Then the value of E(X) is
(A) `37/221`
(B) 5/13
(C) 1/13
(D) 2/13
Assume that the chances of the patient having a heart attack are 40%. It is also assumed that a meditation and yoga course reduce the risk of heart attack by 30% and prescription of certain drug reduces its chances by 25%. At a time a patient can choose any one of the two options with equal probabilities. It is given that after going through one of the two options the patient selected at random suffers a heart attack. Find the probability that the patient followed a course of meditation and yoga?
Two numbers are selected at random (without replacement) from the first five positive integers. Let X denote the larger of the two numbers obtained. Find the mean and variance of X
A random variable X has the following probability distribution:
| Values of X : | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| P (X) : | a | 3a | 5a | 7a | 9a | 11a | 13a | 15a | 17a |
Determine:
(i) The value of a
(ii) P (X < 3), P (X ≥ 3), P (0 < X < 5).
A random variable X takes the values 0, 1, 2 and 3 such that:
P (X = 0) = P (X > 0) = P (X < 0); P (X = −3) = P (X = −2) = P (X = −1); P (X = 1) = P (X = 2) = P (X = 3) . Obtain the probability distribution of X.
A bag contains 4 red and 6 black balls. Three balls are drawn at random. Find the probability distribution of the number of red balls.
Two cards are drawn successively without replacement from a well shuffled pack of 52 cards. Find the probability distribution of the number of aces.
Find the mean and standard deviation of each of the following probability distribution:
| xi : | −1 | 0 | 1 | 2 | 3 |
| pi : | 0.3 | 0.1 | 0.1 | 0.3 | 0.2 |
Find the mean and standard deviation of each of the following probability distribution :
| xi : | -3 | -1 | 0 | 1 | 3 |
| pi : | 0.05 | 0.45 | 0.20 | 0.25 | 0.05 |
Find the mean variance and standard deviation of the following probability distribution
| xi : | a | b |
| pi : | p | q |
A pair of fair dice is thrown. Let X be the random variable which denotes the minimum of the two numbers which appear. Find the probability distribution, mean and variance of X.
A random variable has the following probability distribution:
| X = xi : | 1 | 2 | 3 | 4 |
| P (X = xi) : | k | 2k | 3k | 4k |
Write the value of P (X ≥ 3).
A random variable X has the following probability distribution:
| X : | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| P (X) : | 0.15 | 0.23 | 0.12 | 0.10 | 0.20 | 0.08 | 0.07 | 0.05 |
For the events E = {X : X is a prime number}, F = {X : X < 4}, the probability P (E ∪ F) is
Two fair coins are tossed simultaneously. If X denotes the number of heads, find the probability distribution of X. Also find E(X).
The following data gives the marks of 20 students in mathematics (X) and statistics (Y) each out of 10, expressed as (x, y). construct ungrouped frequency distribution considering single number as a class :
(2, 7) (3, 8) (4, 9) (2, 8) (2, 8) (5, 6) (5 , 7) (4, 9) (3, 8) (4, 8) (2, 9) (3, 8) (4, 8) (5, 6) (4, 7) (4, 7) (4, 6 ) (5, 6) (5, 7 ) (4, 6 )
Verify the following function, which can be regarded as p.m.f. for the given values of X :
| X = x | -1 | 0 | 1 |
| P(x) | -0.2 | 1 | 0.2 |
Find mean and standard deviation of the continuous random variable X whose p.d.f. is given by f(x) = 6x(1 - x);= (0); 0 < x < 1(otherwise)
If X ∼ N (4,25), then find P(x ≤ 4)
Alex spends 20% of his income on food items and 12% on conveyance. If for the month of June 2010, he spent ₹900 on conveyance, find his expenditure on food items during the same month.
Verify whether the following function can be regarded as probability mass function (p.m.f.) for the given values of X :
| X | -1 | 0 | 1 |
| P(X = x) | -0.2 | 1 | 0.2 |
The defects on a plywood sheet occur at random with an average of the defect per 50 sq. ft. What Is the probability that such sheet will have-
(a) No defects
(b) At least one defect
[Use e-1 = 0.3678]
Determine whether each of the following is a probability distribution. Give reasons for your answer.
| y | –1 | 0 | 1 |
| P(y) | 0.6 | 0.1 | 0.2 |
A die is thrown 4 times. If ‘getting an odd number’ is a success, find the probability of at least 3 successes
A die is thrown 4 times. If ‘getting an odd number’ is a success, find the probability of at most 2 successes.
Defects on plywood sheet occur at random with the average of one defect per 50 Sq.ft. Find the probability that such a sheet has no defect
Defects on plywood sheet occur at random with the average of one defect per 50 sq.ft. Find the probability that such a sheet has:
- no defect
- at least one defect
Use e−1 = 0.3678
Solve the following problem :
Following is the probability distribution of a r.v.X.
| x | – 3 | – 2 | –1 | 0 | 1 | 2 | 3 |
| P(X = x) | 0.05 | 0.1 | 0.15 | 0.20 | 0.25 | 0.15 | 0.1 |
Find the probability that X is non-negative
Let a pair of dice be thrown and the random variable X be the sum of the numbers that appear on the two dice. Find the mean or expectation of X and variance of X
Consider the probability distribution of a random variable X:
| X | 0 | 1 | 2 | 3 | 4 |
| P(X) | 0.1 | 0.25 | 0.3 | 0.2 | 0.15 |
Calculate `"V"("X"/2)`
Consider the probability distribution of a random variable X:
| X | 0 | 1 | 2 | 3 | 4 |
| P(X) | 0.1 | 0.25 | 0.3 | 0.2 | 0.15 |
Variance of X.
Two biased dice are thrown together. For the first die P(6) = `1/2`, the other scores being equally likely while for the second die, P(1) = `2/5` and the other scores are equally likely. Find the probability distribution of ‘the number of ones seen’.
The probability distribution of a discrete random variable X is given as under:
| X | 1 | 2 | 4 | 2A | 3A | 5A |
| P(X) | `1/2` | `1/5` | `3/25` | `1/10` | `1/25` | `1/25` |
Calculate: The value of A if E(X) = 2.94
A primary school teacher wants to teach the concept of 'larger number' to the students of Class II.
To teach this concept, he conducts an activity in his class. He asks the children to select two numbers from a set of numbers given as 2, 3, 4, 5 one after the other without replacement.
All the outcomes of this activity are tabulated in the form of ordered pairs given below:
| 2 | 3 | 4 | 5 | |
| 2 | (2, 2) | (2, 3) | (2, 4) | |
| 3 | (3, 2) | (3, 3) | (3, 5) | |
| 4 | (4, 2) | (4, 4) | (4, 5) | |
| 5 | (5, 3) | (5, 4) | (5, 5) |
- Complete the table given above.
- Find the total number of ordered pairs having one larger number.
- Let the random variable X denote the larger of two numbers in the ordered pair.
Now, complete the probability distribution table for X given below.
X 3 4 5 P(X = x) - Find the value of P(X < 5)
- Calculate the expected value of the probability distribution.
Kiran plays a game of throwing a fair die 3 times but to quit as and when she gets a six. Kiran gets +1 point for a six and –1 for any other number.
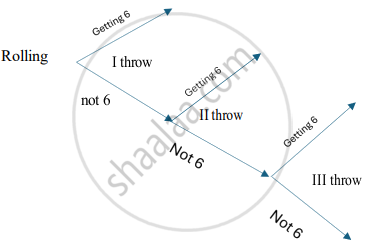
- If X denotes the random variable “points earned” then what are the possible values X can take?
- Find the probability distribution of this random variable X.
- Find the expected value of the points she gets.
