Advertisements
Advertisements
Question
The radii of two circles are 19cm and 9 cm respectively. Find the radius and area of the circle which has circumferences is equal to sum of circumference of two circles.
Solution
Radius of 1st circle = 19cm
Radius of 2nd circle = 9 cm
Circumference of 1st circle = 2(19) = 38𝜋 cm
Circumference of 2nd circle = 2𝜋 (9) = 18𝜋 cm
Let radius of required circle = R cm
Circumference of required circle = 2𝜋R = 𝑐1 + 𝑐2
2𝜋R = 38𝜋 + 18𝜋
2𝜋R = 56𝜋
R = 28 cms
Area of required circle = 𝜋r2
=`22/7× 28 × 28`
= 2464 𝑐𝑚2
APPEARS IN
RELATED QUESTIONS
In fig. 3, a square OABC is inscribed in a quadrant OPBQ of a circle. If OA = 20 cm, find the area of the shaded region. (Use π = 3.14)

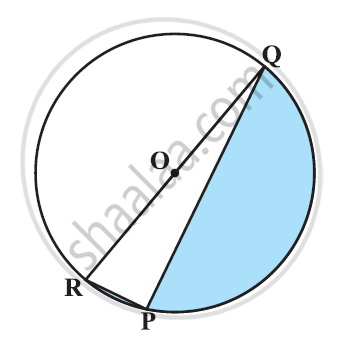
Find the area of the shaded region in the given figure, if PQ = 24 cm, PR = 7 cm and O is the centre of the circle. [Use Π = 22/7]
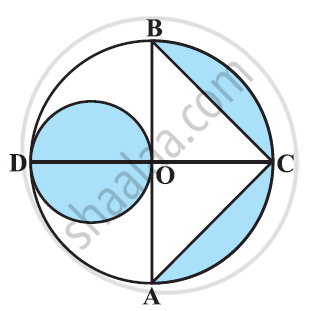
In the given figure, AB and CD are two diameters of a circle (with centre O) perpendicular to each other and OD is the diameter of the smaller circle. If OA = 7 cm, find the area of the shaded region. [Use Π = 22/7]
In the given figure, ABC is a quadrant of a circle of radius 14 cm and a semicircle is drawn with BC as diameter. Find the area of the shaded region. [Use Π = 22/7]

Calculate the area of the designed region in the given figure common between the two quadrants of circles of radius 8 cm each. [Use Π = 22/7]

The difference between the sides at the right angles in a right-angled triangle is 7 cm. the area of the triangle is `60 cm^2` . Find its perimeter.
The height of an equilateral triangle is 6 cm. Find its area.
If the area of an equilateral triangle is `36sqrt3 cm^2` find its perimeter.
Find the perimeter of the shaded region in Figure 4, if ABCD is a square of side 14 cm and APB and CPD are semicircles. Use `pi = 22/7`
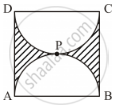
Read the following passage:
|
For the inauguration of 'Earth day' week in a school, badges were given to volunteers. Organisers purchased these badges from an NGO, who made these badges in the form of a circle inscribed in a square of side 8 cm.
|
Based on the above information, answer the following questions:
- What is the area of square ABCD?
- What is the length of diagonal AC of square ABCD?
- Find the area of sector OPRQO.
OR
Find the area of remaining part of square ABCD when area of circle is excluded.


