Advertisements
Advertisements
Question
Two supplementary angles are in the ratio 4 : 5. Find the angles.
Solution
Supplementary angles are in the ratio 4 : 5
Let the angles be 4x and 5x
It is given that they are supplementary angles
∴ 4x + 5x = 180°x
⇒ 9x = 180°
⇒ x = 20°
Hence, 4x = 4 (20) = 80°
5( x) = 5(20) = 100°
∴ Angles are 80° and 100°
APPEARS IN
RELATED QUESTIONS
Write the complement of the following angles .
35°
If the angles (2x −10)° and (x − 5)° are complementary angles, find x.
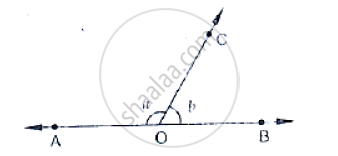
In the below Fig, ∠AOC and ∠BOC form a linear pair. if a − 2b = 30°, find a and b.
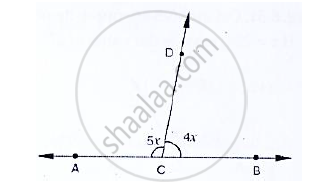
In the below fig, ACB is a line such that ∠DCA = 5x and ∠DCB = 4x. Find the value of x.
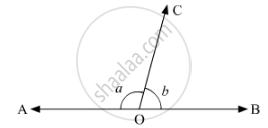
In Fig. 8.42, a is greater than b by one third of a right-angle. Find the values of a and b.
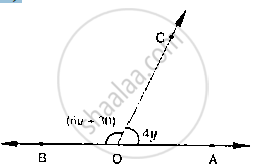
What value of y would make AOB a line in below fig, if ∠AOC = 4y and ∠BOC = (6y +
30)
statement are true and false
Angles forming a linear pair are supplementary.
The opposite sides of a quadrilateral are parallel. If one angle of the quadrilateral is 60°,
find the other angles.
In the given figure, if l1 || l2, what is the value of x?

If two lines intersect, prove that the vertically opposite angles are equal.
