Advertisements
Advertisements
Question
Using Ampere’s circuital law, obtain the expression for the magnetic field due to a long solenoid at a point inside the solenoid on its axis ?
Solution
Solenoid
• It consists of an insulating long wire closely wound in the form of helix.
• Its length is large as compared to its diameter.
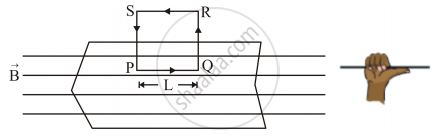
• Magnetic field due to RQ and SP path is zero because they are perpendicular to the axis of solenoid. Since SR is outside the solenoid, the magnetic field is zero.
• The line integral of magnetic field induction `vecB` over the closed path PQRS is
`oint_(PQRS) vecB*vecdl =oint_(PQ)vecB*vecdl =BL`
From Ampere’s circuital law,
`oint_(PQRS)vecB*vecdl = mu_0 `× Total current through rectangle PQRS
BL = μ0 × Number of turns in rectangle × Current
BL = μ0nLI
∴ B= μ0nI
APPEARS IN
RELATED QUESTIONS
A long, straight wire carries a current. Is Ampere's law valid for a loop that does not enclose the wire, or that encloses the wire but is not circular?
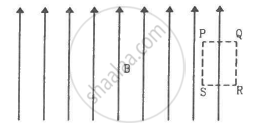
Sometimes we show an idealised magnetic field which is uniform in a given region and falls to zero abruptly. One such field is represented in figure. Using Ampere's law over the path PQRS, show that such a field is not possible.
What is magnetic permeability?
Define ampere.
A straight wire of diameter 0.5 mm carrying a current of 1 A is replaced by another wire of 1 mm diameter carrying the same current. The strength of the magnetic field far away is ______.
A long solenoid having 200 turns per cm carries a current of 1.5 amp. At the centre of it is placed a coil of 100 turns of cross-sectional area 3.14 × 10−4 m2 having its axis parallel to the field produced by the solenoid. When the direction of current in the solenoid is reversed within 0.05 sec, the induced e.m.f. in the coil is:
Two identical current carrying coaxial loops, carry current I in an opposite sense. A simple amperian loop passes through both of them once. Calling the loop as C ______.
- `oint B.dl = +- 2μ_0I`
- the value of `oint B.dl` is independent of sense of C.
- there may be a point on C where B and dl are perpendicular.
- B vanishes everywhere on C.
Read the following paragraph and answer the questions.
|
Consider the experimental set-up shown in the figure. This jumping ring experiment is an outstanding demonstration of some simple laws of Physics. A conducting non-magnetic ring is placed over the vertical core of a solenoid. When current is passed through the solenoid, the ring is thrown off. |
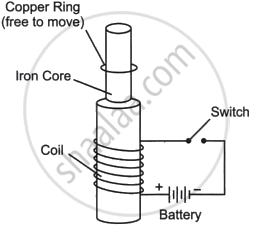
- Explain the reason for the jumping of the ring when the switch is closed in the circuit.
- What will happen if the terminals of the battery are reversed and the switch is closed? Explain.
- Explain the two laws that help us understand this phenomenon.
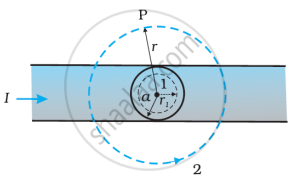
The given figure shows a long straight wire of a circular cross-section (radius a) carrying steady current I. The current I is uniformly distributed across this cross-section. Calculate the magnetic field in the region r < a and r > a.
Briefly explain various ways to increase the strength of the magnetic field produced by a given solenoid.
