Advertisements
Advertisements
Question
Read the following paragraph and answer the questions.
|
Consider the experimental set-up shown in the figure. This jumping ring experiment is an outstanding demonstration of some simple laws of Physics. A conducting non-magnetic ring is placed over the vertical core of a solenoid. When current is passed through the solenoid, the ring is thrown off. |
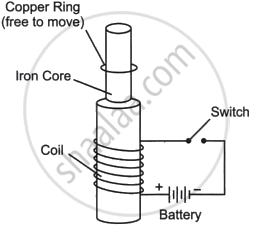
- Explain the reason for the jumping of the ring when the switch is closed in the circuit.
- What will happen if the terminals of the battery are reversed and the switch is closed? Explain.
- Explain the two laws that help us understand this phenomenon.
Solution
- When a switch in a circuit is closed, current flows, causing emf in the circuit. The magnetic field of the solenoid varies continually due to ac. This causes an eddy current to form in the ring. The magnetic field produced by the induced eddy current in the ring, according to Lenz's law, opposes the changing magnetic field of the solenoid. As a result, the two magnetic fields repel one another, causing the ring to leap.
- When the switch is closed and the battery terminals are reversed, the magnetic field is reversed as well because the direction of the current is likewise reversed.
- The two laws that assist us in understanding this phenomenon are Faraday's laws and Lenz's law of electromagnetic induction.
When the induced current is applied in the same direction as the coil, the motion is countered by Lenz's law. The magnet in the loop exerts force to generate the current. To counteract the change, the magnet's current must impose a force on it.
"Whenever a conductor is placed in a varying magnetic field, an electromotive force is induced," asserts Faraday's first law of electromagnetic induction. Similarly, when the conductor circuit is closed, a current is induced, which is referred to as induced current."
APPEARS IN
RELATED QUESTIONS
Write Maxwell's generalization of Ampere's circuital law. Show that in the process of charging a capacitor, the current produced within the plates of the capacitor is `I=varepsilon_0 (dphi_E)/dt,`where ΦE is the electric flux produced during charging of the capacitor plates.
A long, cylindrical wire of radius b carries a current i distributed uniformly over its cross section. Find the magnitude of the magnetic field at a point inside the wire at a distance a from the axis.
A solid wire of radius 10 cm carries a current of 5.0 A distributed uniformly over its cross section. Find the magnetic field B at a point at a distance (a) 2 cm (b) 10 cm and (c) 20 cm away from the axis. Sketch a graph B versus x for 0 < x < 20 cm.
Using Ampere's circuital law, obtain an expression for the magnetic flux density 'B' at a point 'X' at a perpendicular distance 'r' from a long current-carrying conductor.
(Statement of the law is not required).
What is magnetic permeability?
Define ampere.
The force required to double the length of a steel wire of area 1 cm2, if it's Young's modulus Y = `2 xx 10^11/m^2` is:
A thick current carrying cable of radius ‘R’ carries current ‘I’ uniformly distributed across its cross-section. The variation of magnetic field B(r) due to the cable with the distance ‘r’ from the axis of the cable is represented by:
Two identical current carrying coaxial loops, carry current I in an opposite sense. A simple amperian loop passes through both of them once. Calling the loop as C ______.
- `oint B.dl = +- 2μ_0I`
- the value of `oint B.dl` is independent of sense of C.
- there may be a point on C where B and dl are perpendicular.
- B vanishes everywhere on C.
Briefly explain various ways to increase the strength of the magnetic field produced by a given solenoid.
