Advertisements
Advertisements
Question
Which of the following relations are functions? If it is a function determine its domain and range:
{(1, 1), (3, 1), (5, 2)}
Solution
{(1, 1), (3, 1), (5, 2)}
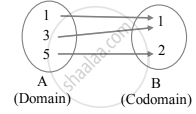
Every element of set A has been assigned a unique element in set B.
∴ Given relation is a function.
Domain = {1, 3, 5}, Range = {1, 2}
APPEARS IN
RELATED QUESTIONS
If f(x) = x2, find `(f(1.1) - f(1))/((1.1 - 1))`
Let f : R → R and g : C → C be two functions defined as f(x) = x2 and g(x) = x2. Are they equal functions?
If \[f\left( x \right) = \frac{1}{1 - x}\] , show that f[f[f(x)]] = x.
If for non-zero x, af(x) + bf \[\left( \frac{1}{x} \right) = \frac{1}{x} - 5\] , where a ≠ b, then find f(x).
Write the range of the function f(x) = cos [x], where \[\frac{- \pi}{2} < x < \frac{\pi}{2}\] .
If f(x) = cos (log x), then the value of f(x) f(y) −\[\frac{1}{2}\left\{ f\left( \frac{x}{y} \right) + f\left( xy \right) \right\}\] is
If f(m) = m2 − 3m + 1, find f(−3)
Which sets of ordered pairs represent functions from A = {1, 2, 3, 4} to B = {−1, 0, 1, 2, 3}? Justify.
{(1, 3), (4, 1), (2, 2)}
Find x, if g(x) = 0 where g(x) = x3 − 2x2 − 5x + 6
Find x, if f(x) = g(x) where f(x) = x4 + 2x2, g(x) = 11x2
Find the domain and range of the following function.
f(x) = `root(3)(x + 1)`
Express the following logarithmic equation in exponential form
`log_5 1/25` = – 2
Express the following logarithmic equation in exponential form
ln 1 = 0
Answer the following:
Simplify `log_10 28/45 - log_10 35/324 + log_10 325/432 - log_10 13/15`
Find the domain of the following function.
f(x) = `sqrtlog(x^2 - 6x + 6)`
Answer the following:
Find the range of the following function.
f(x) = `x/(9 + x^2)`
A function f is defined by f(x) = 2x – 3 find x such that f(x) = x
The domain of the function f defined by f(x) = `1/sqrt(x - |x|)` is ______.
Let f(x) = `sqrt(1 + x^2)`, then ______.
The domain of the function f(x) = `sin^-1((|x| + 5)/(x^2 + 1))` is (–∞, –a] ≈ [a, ∞). Then a is equal to ______.
