Advertisements
Advertisements
Question
Write the domain and range of function f(x) given by \[f\left( x \right) = \sqrt{\left[ x \right] - x}\] .
Solution
\[\text{ We know that } \]
\[[x] - x = - {x}, \text{ which is the fractional part of any real number x } . \]
\[\text{ Thus } , f(x) = \sqrt{- {x}} . \]
\[\text{ Since {x} is always a positive number, f(x) is not defined for any x . } \]
\[\text{ Or dom } (f) = \varphi\]
\[\text{ Thus, range } (f) = \varphi . \]
APPEARS IN
RELATED QUESTIONS
Let f be the subset of Z × Z defined by f = {(ab, a + b): a, b ∈ Z}. Is f a function from Z to Z: justify your answer.
Let f : R+ → R, where R+ is the set of all positive real numbers, such that f(x) = loge x. Determine
(a) the image set of the domain of f
Let X = {1, 2, 3, 4} and Y = {1, 5, 9, 11, 15, 16}
Determine which of the set are functions from X to Y.
(c) f3 = {(1, 5), (2, 9), (3, 1), (4, 5), (2, 11)}
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(i) f + g
If \[f\left( x \right) = \log \left( \frac{1 + x}{1 - x} \right)\] , then \[f\left( \frac{2x}{1 + x^2} \right)\] is equal to
If \[f\left( x \right) = \frac{\sin^4 x + \cos^2 x}{\sin^2 x + \cos^4 x}\] for x ∈ R, then f (2002) =
Let A = {x ∈ R : x ≠ 0, −4 ≤ x ≤ 4} and f : A ∈ R be defined by \[f\left( x \right) = \frac{\left| x \right|}{x}\] for x ∈ A. Then th (is
The domain of definition of the function \[f\left( x \right) = \sqrt{\frac{x - 2}{x + 2}} + \sqrt{\frac{1 - x}{1 + x}}\] is
Check if the following relation is function:
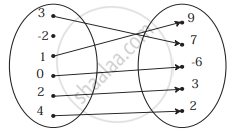
A function f is defined as follows: f(x) = 4x + 5, for −4 ≤ x < 0. Find the values of f(−1), f(−2), f(0), if they exist.
If f(m) = m2 − 3m + 1, find f(0)
Find x, if g(x) = 0 where g(x) = 6x2 + x − 2
Find x, if g(x) = 0 where g(x) = x3 − 2x2 − 5x + 6
Find the domain and range of the following function.
f(x) = `root(3)(x + 1)`
Find the domain and range of the following function.
f(x) = `sqrt((x - 3)/(7 - x))`
Express the area A of circle as a function of its radius r
Express the area A of circle as a function of its circumference C.
lf f(x) = 3(4x+1), find f(– 3)
Express the following logarithmic equation in exponential form
log2 64 = 6
Express the following logarithmic equation in exponential form
log10 (0.001) = −3
Write the following expression as sum or difference of logarithm
In `(("a"^3 ("a" - 2)^2)/sqrt("b"^2 + 5))`
Prove that logbm a = `1/"m" log_"b""a"`
Answer the following:
Solve for x, logx (8x – 3) – logx 4 = 2
Answer the following:
If a2 + b2 = 7ab, show that, `log(("a" + "b")/3) = 1/2 log "a" + 1/2 log "b"`
Answer the following:
If `log ((x - y)/5) = 1/2 logx + 1/2 log y`, show that x2 + y2 = 27xy
Answer the following:
Without using log tables, prove that `2/5 < log_10 3 < 1/2`
Answer the following:
If `log"a"/(x + y - 2z) = log"b"/(y + z - 2x) = log"c"/(z + x - 2y)`, show that abc = 1
A plane is flying at a speed of 500 km per hour. Express the distance ‘d’ travelled by the plane as function of time t in hour
The range of the function f(x) = `(x - 3)/(5 - x)`, x ≠ 5 is ______.
Domain of function f(x) = cos–1 6x is ______.
If f(x) = `1/sqrt(4 - 3x)`, then dom(f) = ______..
Find the range of the following functions given by f(x) = 1 – |x – 2|
If f(x) = `(x - 1)/(x + 1)`, then show that `f(- 1/x) = (-1)/(f(x))`
The domain of the function f defined by f(x) = `sqrt(4 - x) + 1/sqrt(x^2 - 1)` is equal to ______.
The function f: R `rightarrow` R defined by f(x) = sin x is ______.
If f: R `rightarrow` R be a function defined by f(x) = 4x3 – 7. Then ______.
Range of the function f(x) = `x/(1 + x^2)` is ______.
