Advertisements
Advertisements
Question
lf f(x) = 3(4x+1), find f(– 3)
Solution
f(x) = 3(4x+1)
∴ f(– 3) = 3(4–3+1)
= `3/(4^(-2))`
= `3/16`.
APPEARS IN
RELATED QUESTIONS
Define a function as a correspondence between two sets.
find: f(1), f(−1), f(0) and f(2).
Let X = {1, 2, 3, 4} and Y = {1, 5, 9, 11, 15, 16}
Determine which of the set are functions from X to Y.
(a) f1 = {(1, 1), (2, 11), (3, 1), (4, 15)}
If f : R → R be defined by f(x) = x2 + 1, then find f−1 [17] and f−1 [−3].
Let A = [p, q, r, s] and B = [1, 2, 3]. Which of the following relations from A to B is not a function?
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(ii) g − f
If f : [−2, 2] → R is defined by \[f\left( x \right) = \begin{cases}- 1, & \text{ for } - 2 \leq x \leq 0 \\ x - 1, & \text{ for } 0 \leq x \leq 2\end{cases}\] , then
{x ∈ [−2, 2] : x ≤ 0 and f (|x|) = x} =
If \[e^{f\left( x \right)} = \frac{10 + x}{10 - x}\] , x ∈ (−10, 10) and \[f\left( x \right) = kf\left( \frac{200 x}{100 + x^2} \right)\] , then k =
The domain of definition of \[f\left( x \right) = \sqrt{x - 3 - 2\sqrt{x - 4}} - \sqrt{x - 3 + 2\sqrt{x - 4}}\] is
The range of the function \[f\left( x \right) = \frac{x}{\left| x \right|}\] is
Let \[f\left( x \right) = \sqrt{x^2 + 1}\ ] . Then, which of the following is correct?
If \[\left[ x \right]^2 - 5\left[ x \right] + 6 = 0\], where [.] denotes the greatest integer function, then
Check if the following relation is function:

If f(x) = `{(x^2 + 3"," x ≤ 2),(5x + 7"," x > 2):},` then find f(3)
Which of the following relations are functions? If it is a function determine its domain and range:
{(2, 1), (4, 2), (6, 3), (8, 4), (10, 5), (12, 6), (14, 7)}
If f(x) = ax2 + bx + 2 and f(1) = 3, f(4) = 42, find a and b.
Which sets of ordered pairs represent functions from A = {1, 2, 3, 4} to B = {−1, 0, 1, 2, 3}? Justify.
{(1, 1), (2, 1), (3, 1), (4, 1)}
Find x, if g(x) = 0 where g(x) = 6x2 + x − 2
Find the domain and range of the following function.
g(x) = `(x + 4)/(x - 2)`
Find the domain and range of the following function.
f(x) = `sqrt((x - 2)(5 - x)`
Express the area A of circle as a function of its diameter d
Check the injectivity and surjectivity of the following function.
f : N → N given by f(x) = x2
Express the following logarithmic equation in exponential form
log2 64 = 6
Find the domain of f(x) = ln (x − 5)
A function f is defined as : f(x) = 5 – x for 0 ≤ x ≤ 4. Find the value of x such that f(x) = 3
Answer the following:
Find x, if x = 33log32
Answer the following:
Find the range of the following function.
f(x) = |x – 5|
A graph representing the function f(x) is given in it is clear that f(9) = 2
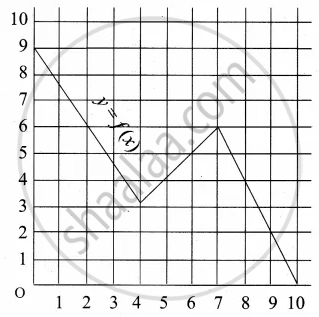
For what value of x is f(x) = 1?
Let f(x) = 2x + 5. If x ≠ 0 then find `(f(x + 2) -"f"(2))/x`
A plane is flying at a speed of 500 km per hour. Express the distance ‘d’ travelled by the plane as function of time t in hour
If f(x) = 5x - 3, then f-1(x) is ______
Mapping f: R → R which is defined as f(x) = sin x, x ∈ R will be ______
Domain of function f(x) = cos–1 6x is ______.
If f(x) = `x^3 - 1/x^3`, then `f(x) + f(1/x)` is equal to ______.
Let f(x) = `sqrt(x)` and g(x) = x be two functions defined in the domain R+ ∪ {0}. Find (fg)(x)
If f(x) = `log_e{((1 - x))/((1 - x))}, |x| < 1, f{(2x)/((1 + x^2))}` is equal to ______.
If f: R `rightarrow` R be a function defined by f(x) = 4x3 – 7. Then ______.
If f : R – {2} `rightarrow` R i s a function defined by f(x) = `(x^2 - 4)/(x - 2)`, then its range is ______.
The domain of f(x) = `sin^-1 [log_2(x/2)]` is ______.
