Advertisements
Advertisements
Question
The domain of definition of the function \[f\left( x \right) = \sqrt{\frac{x - 2}{x + 2}} + \sqrt{\frac{1 - x}{1 + x}}\] is
Options
(a) (−∞, −2] ∪ [2, ∞)
(b) [−1, 1]
(c) ϕ
(d) None of these
Solution
(c) ϕ
\[\text{ For f(x) to be defined,} \]
\[x + 2 \neq 0\]
\[ \Rightarrow x \neq - 2 . . . (1)\]
\[\text{ And } 1 + x \neq 0\]
\[ \Rightarrow x \neq - 1 . . . . (2)\]
\[\text{ Also } , \frac{x - 2}{x + 2} \geq 0\]
\[ \Rightarrow \frac{(x - 2)(x + 2)}{(x + 2 )^2} \geq 0\]
\[ \Rightarrow (x - 2)(x + 2) \geq 0\]
\[ \Rightarrow x \in ( - \infty , - 2) \cup [2, \infty ) . . . (3)\]
\[\text{ And } \frac{1 - x}{1 + x} \geq 0\]
\[ \Rightarrow \frac{(1 - x)(1 + x)}{(1 + x )^2} \geq 0\]
\[ \Rightarrow (1 - x)(1 + x) \geq 0\]
\[ \Rightarrow x \in ( - \infty , - 1) \cup [1, \infty ) . . . (4)\]
\[\text{ From (1), (2), (3) and (4), we get,} \]
\[x \in \phi . \]
\[\text{ Thus, dom } (f(x)) = \phi . \]
APPEARS IN
RELATED QUESTIONS
Find the domain of the function f(x) = `(x^2 + 2x + 1)/(x^2 - 8x + 12)`
Let f : R+ → R, where R+ is the set of all positive real numbers, such that f(x) = loge x. Determine
(c) whether f(xy) = f(x) : f(y) holds
Let A = [p, q, r, s] and B = [1, 2, 3]. Which of the following relations from A to B is not a function?
Let f and g be two real functions defined by \[f\left( x \right) = \sqrt{x + 1}\] and \[g\left( x \right) = \sqrt{9 - x^2}\] . Then, describe function:
(iii) f g
Write the range of the function f(x) = cos [x], where \[\frac{- \pi}{2} < x < \frac{\pi}{2}\] .
Write the range of the function f(x) = ex−[x], x ∈ R.
Let \[f\left( x \right) = \frac{\alpha x}{x + 1}, x \neq - 1\] . Then write the value of α satisfying f(f(x)) = x for all x ≠ −1.
Write the domain and range of function f(x) given by \[f\left( x \right) = \sqrt{\left[ x \right] - x}\] .
Let f and g be two functions given by
f = {(2, 4), (5, 6), (8, −1), (10, −3)} and g = {(2, 5), (7, 1), (8, 4), (10, 13), (11, −5)}.
Find the domain of f + g
If \[f\left( x \right) = \frac{2^x + 2^{- x}}{2}\] , then f(x + y) f(x − y) is equal to
If f(x) = cos (loge x), then \[f\left( \frac{1}{x} \right)f\left( \frac{1}{y} \right) - \frac{1}{2}\left\{ f\left( xy \right) + f\left( \frac{x}{y} \right) \right\}\] is equal to
If f(x) = sin [π2] x + sin [−π]2 x, where [x] denotes the greatest integer less than or equal to x, then
A function f is defined as follows: f(x) = 5 − x for 0 ≤ x ≤ 4. Find the value of x such that f(x) = 3
Check if the following relation is a function.
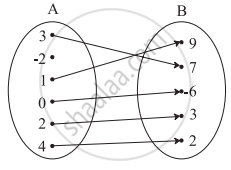
Which sets of ordered pairs represent functions from A = {1, 2, 3, 4} to B = {−1, 0, 1, 2, 3}? Justify.
{(1, 1), (2, 1), (3, 1), (4, 1)}
If f(x) = `("a" - x)/("b" - x)`, f(2) is undefined, and f(3) = 5, find a and b
Find the domain and range of the following function.
f(x) = `root(3)(x + 1)`
Write the following expression as sum or difference of logarithm
`log (sqrt(x) root(3)(y))`
Write the following expression as sum or difference of logarithm
In `(("a"^3 ("a" - 2)^2)/sqrt("b"^2 + 5))`
Write the following expression as a single logarithm.
5 log x + 7 log y − log z
Solve for x.
2 log10 x = `1 + log_10 (x + 11/10)`
Select the correct answer from given alternatives.
If log (5x – 9) – log (x + 3) = log 2 then x = ...............
Answer the following:
Find whether the following function is one-one
f : R − {3} → R defined by f(x) = `(5x + 7)/(x - 3)` for x ∈ R − {3}
Answer the following:
Let f : R → R be given by f(x) = x3 + 1 for all x ∈ R. Draw its graph
Answer the following:
Find value of `(3 + log_10 343)/(2 + 1/2 log_10 (49/4) + 1/2 log_10 (1/25)`
Answer the following:
If `log"a"/(x + y - 2z) = log"b"/(y + z - 2x) = log"c"/(z + x - 2y)`, show that abc = 1
Let X = {3, 4, 6, 8}. Determine whether the relation R = {(x, f(x)) | x ∈ X, f(x) = x2 + 1} is a function from X to N?
Given the function f: x → x2 – 5x + 6, evaluate f(2a)
Given the function f: x → x2 – 5x + 6, evaluate f(x – 1)
A graph representing the function f(x) is given in it is clear that f(9) = 2
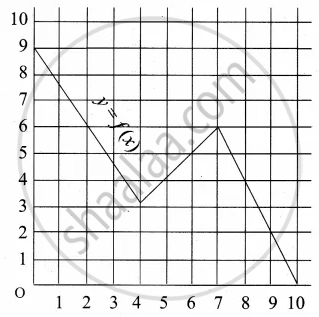
Find the following values of the function
(a) f(0)
(b) f(7)
(c) f(2)
(d) f(10)
A graph representing the function f(x) is given in it is clear that f(9) = 2
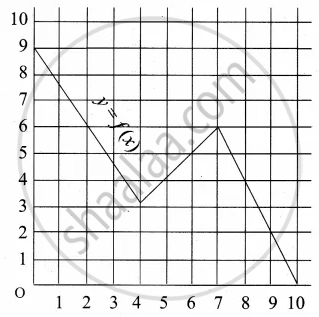
Describe the following Domain
The data in the adjacent table depicts the length of a person's forehand and their corresponding height. Based on this data, a student finds a relationship between the height (y) and the forehand length (x) as y = ax + b, where a, b are constant.
| Length ‘x’ of forehand (in cm) |
Height 'y' (in inches) |
| 35 | 56 |
| 45 | 65 |
| 50 | 69.5 |
| 55 | 74 |
Find the length of forehand of a person if the height is 53.3 inches
The function f and g are defined by f(x) = 6x + 8; g(x) = `(x - 2)/3`
Calculate the value of `"gg" (1/2)`
The range of 7, 11, 16, 27, 31, 33, 42, 49 is ______.
Mapping f: R → R which is defined as f(x) = sin x, x ∈ R will be ______
If f(x) = `1/sqrt(4 - 3x)`, then dom(f) = ______..
Find the domain of the following functions given by f(x) = `1/sqrt(1 - cos x)`
If f(x) = `(x - 1)/(x + 1)`, then show that `f(- 1/x) = (-1)/(f(x))`
If f(x) = x3 – 1 and domain of f = {0, 1, 2, 3}, then domain of f–1 is ______.
