(English Medium)
Academic Year: 2022-2023
Date & Time: 10th February 2023, 11:00 am
Duration: 2h30m
Advertisements
- Answers to this Paper must be written on the paper provided separately.
- You will not be allowed to write during the first 15 minutes.
- This time is to be spent reading the question paper.
- The time given at the head of this Paper is the time allowed for writing the answers.
- Attempt all questions from Section A and any four questions from Section B.
- All work, including rough work, must be clearly shown and must be done on the same sheet as the rest of the Solution.
- Omission of essential work will result in a loss of marks.
- The intended marks for questions or parts of questions are given in brackets [ ].
- Mathematical tables and graph papers are provided.
If `[(2, 0),(0, 4)][(x),(y)] = [(2),(-8)]`, the value of x and y respectively are ______.
1, –2
–2, 1
1, 2
–2, –1
Chapter: [0.09] Matrices
If x – 2 is a factor of x3 – kx – 12, then the value of k is ______.
3
2
–2
–3
Chapter: [0.08] Factorization
In the given diagram RT is a tangent touching the circle at S. If ∠PST = 30° and ∠SPQ = 60°, then ∠PSQ is equal to ______.
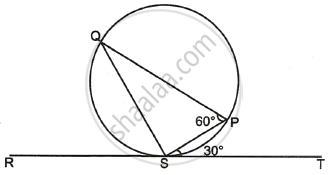
40°
30°
60°
90°
Chapter: [0.17] Circles
A letter is chosen at random from all the letters of the English alphabets. The probability that the letter chosen is a vowel, is ______.
`4/26`
`5/26`
`21/26`
`5/24`
Chapter: [0.07] Probability
If 3 is a root of the quadratic equation x2 – px + 3 = 0 then p is equal to ______.
4
3
5
2
Chapter: [0.05] Quadratic Equations
In the given figure ∠BAP = ∠DCP = 70°, PC = 6 cm and CA = 4 cm, then PD : DB is ______.
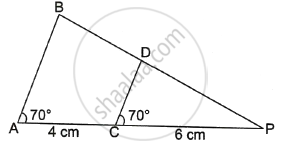
5 : 3
3 : 5
3 : 2
2 : 3
Chapter: [0.15] Similarity
The printed price of an article is ₹ 3080. If the rate of GST is 10%, then the GST charged is ______.
₹ 154
₹ 308
₹ 30.80
₹ 15.40
Chapter: [0.01] GST (Goods and Services Tax)
(1 + sin A)(1 – sin A) is equal to ______.
cosec2A
sin2A
sec2A
cos2A
Chapter: [0.05] Trigonometry
The coordinates of the vertices of ΔABC are respectively (–4, –2), (6, 2) and (4, 6). The centroid G of ΔABC is ______.
(2, 2)
(2, 3)
(3, 3)
(0, –1)
Chapter: [0.13] Co-ordinate Geometry Distance and Section Formula
The nth term of an Arithmetic Progression (A.P.) is 2n + 5. The 10th term is ______.
7
15
25
45
Chapter: [0.1] Arithmetic Progression
The mean proportional between 4 and 9 is ______.
4
6
9
36
Chapter: [0.07] Ratio and Proportion
Which of the following cannot be determined graphically for a grouped frequency distribution?
Median
Mode
Quartiles
Mean
Chapter: [0.06] Statistics
Volume of a cylinder of height 3 cm is a 48π. Radius of the cylinder is ______.
48 cm
16 cm
4 cm
24 cm
Chapter: [0.04] Mensuration
Naveen deposits ₹ 800 every month in a recurring deposit account for 6 months. If he receives ₹ 4884 at the time of maturity, then the interest he earns is ______.
₹ 84
₹ 42
₹ 24
₹ 284
Chapter: [0.02] Banking
The solution set for the inequation 2x + 4 ≤ 14, x ∈ W is ______.
{1, 2, 3, 4, 5}
{0, 1, 2, 3, 4, 5}
{1, 2, 3, 4}
{0, 1, 2, 3, 4}
Chapter: [0.04] Linear Inequations
Advertisements
Find the value of 'a' if x – a is a factor of the polynomial 3x3 + x2 – ax – 81.
Chapter: [0.08] Factorization
Salman deposits ₹ 1000 every month in a recurring deposit account for 2 years. If he receives ₹ 26000 on maturity, find:
- the total interest Salman earns.
- the rate of interest.
Chapter: [0.02] Banking
In the given figure O, is the centre of the circle. CE is a tangent to the circle at A. If ∠ABD = 26° find:
- ∠BDA
- ∠BAD
- ∠CAD
- ∠ODB

Chapter: [0.17] Circles
Solve the following quadratic equation:
x2 + 4x – 8 = 0
Give your Solution correct to one decimal place.
(Use mathematical tables if necessary.)
Chapter: [0.05] Quadratic Equations
Prove the following identity:
(sin2θ – 1)(tan2θ + 1) + 1 = 0
Chapter: [0.05] Trigonometry
Use graph sheet to Solution this question. Take 2 cm = 1 unit alogn both the axes.
- Plot A, B, C where A(0, 4), B(1, 1) and C(4, 0)
- Reflect A and B on the x-axis and name them as E and D respectively.
- Reflect B through the origion and name it F. Write down the coordinates of F.
- Reflect B and C on the y-axis and name them as H and G respectively.
- Join points A, B, C, D, E, F, G, H and A in order and name the closed figure formed.
Chapter: [0.12] Reflection
If A = `[(1, 3),(2, 4)]`, B = `[(1, 2),(2, 4)]`, C = `[(4, 1),(1, 5)]` and I = `[(1, 0),(0, 1)]`. Find A(B + C) – 14I.
Chapter: [0.09] Matrices
ABC is a triangle whose vertices are A(1, –1), B(0, 4) and C(– 6, 4). D is the midpoint of BC. Find the coordinates of D.
Chapter: [0.13] Co-ordinate Geometry Distance and Section Formula
ABC is a triangle whose vertices are A(1, –1), B(0, 4) and C(– 6, 4). D is the midpoint of BC. Find the equation of the median AD.
Chapter: [0.14] Co-ordinate Geometry Equation of a Line
In the given figure, O is the centre of the circle. PQ is a tangent to the circle at T. Chord AB produced meets the tangent at P.
AB = 9 cm, BP = 16 cm, ∠PTB = 50° ∠OBA = 45°
Find:
- Length of PT
- ∠BAT
- ∠BOT
- ∠ABT
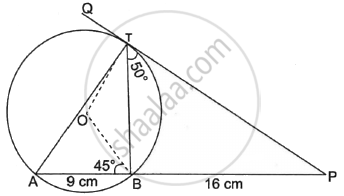
Chapter: [0.17] Circles
Mrs. Arora bought the following articles from a departmental store:
| S.No. | Item | Price | Rate of GST | Discount |
| 1. | Hair oil | ₹ 1200 | 18% | ₹ 100 |
| 2. | Cashew nuts | ₹ 600 | 12% |
Find the:
- Total GST paid,
- Total bill amount including GST.
Chapter: [0.01] GST (Goods and Services Tax)
Solve the following inequation. Write down the solution set and represent it on the real number line.
–5(x – 9) ≥ 17 – 9x > x + 2, x ∈ R
Chapter: [0.04] Linear Inequations
In the given figure, AC || DE || BF. If AC = 24 cm, EG = 8 cm, GB = 16 cm, BF = 30 cm.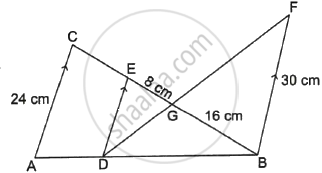
- Prove ΔGED ∼ ΔGBF
- Find DE
- DB : AB
Chapter: [0.15] Similarity
The following distribution gives the daily wages of 60 workers of a factory.
| Daily income in ₹ |
Number of workers (f) |
| 200 – 300 | 6 |
| 300 – 400 | 10 |
| 400 – 500 | 14 |
| 500 – 600 | 16 |
| 600 – 700 | 10 |
| 700 – 800 | 4 |
Use graph paper to Solution this question.
Take 2 cm = ₹ 100 along one axis and 2 cm = 2 workers along the other axis.
Draw a histogram and hence find the mode of the given distribution.
Chapter: [0.06] Statistics
Advertisements
The 5th term and the 9th term of an Arithmetic Progression are 4 and – 12 respectively.
Find:
- the first term
- common difference
- sum of 16 terms of the AP.
Chapter: [0.1] Arithmetic Progression
A and B are two points on the x-axis and y-axis respectively.

- Write down the coordinates of A and B.
- P is a point on AB such that AP : PB = 1 : 1.
Using section formula find the coordinates of point P. - Find the equation of a line passing through P and perpendicular to AB.
Chapter: [0.14] Co-ordinate Geometry Equation of a Line
A bag contains 25 cards, numbered through 1 to 25. A card is drawn at random. What is the probability that the number on the card drawn is multiple of 5?
Chapter: [0.07] Probability
A bag contains 25 cards, numbered through 1 to 25. A card is drawn at random. What is the probability that the number on the card drawn is a perfect square?
Chapter: [0.07] Probability
A bag contains 25 cards, numbered through 1 to 25. A card is drawn at random. What is the probability that the number on the card drawn is a prime number?
Chapter: [0.07] Probability
A man covers a distance of 100 km, travelling with a uniform speed of x km/hr. Had the speed been 5 km/hr more it would have taken 1 hour less. Find x the original speed.
Chapter: [0.06] Solving (Simple) Problems (Based on Quadratic Equations)
A solid is in the shape of a hemisphere of radius 7 cm, surmounted by a cone of height 4 cm. The solid is immersed completely in a cylindrical container filled with water to a certain height. If the radius of the cylinder is 14 cm, find the rise in the water level.
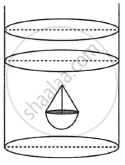
Chapter: [0.04] Mensuration
The following table gives the marks scored by a set of students in an examination. Calculate the mean of the distribution by using the short cut method.
| Marks | Number of Students (f) |
| 0 – 10 | 3 |
| 10 – 20 | 8 |
| 20 – 30 | 14 |
| 30 – 40 | 9 |
| 40 – 50 | 4 |
| 50 – 60 | 2 |
Chapter: [0.06] Statistics
What number must be added to each of the numbers 4, 6, 8, 11 in order to get the four numbers in proportion?
Chapter: [0.07] Ratio and Proportion
Using ruler and compass construct a triangle ABC in which AB = 6 cm, ∠BAC = 120° and AC = 5 cm. Construct a circle passing through A, B and C. Measure and write down the radius of the circle.
Chapter: [0.18] Constructions
Using Componendo and Dividendo solve for x:
`(sqrt(2x + 2) + sqrt(2x - 1))/(sqrt(2x + 2) - sqrt(2x - 1))` = 3
Chapter: [0.07] Ratio and Proportion
Which term of the Arithmetic Progression (A.P.) 15, 30, 45, 60...... is 300?
Hence find the sum of all the terms of the Arithmetic Progression (A.P.)
Chapter: [0.1] Arithmetic Progression
From the top of a tower 100 m high a man observes the angles of depression of two ships A and B, on opposite sides of the tower as 45° and 38° respectively. If the foot of the tower and the ships are in the same horizontal line find the distance between the two ships A and B to the nearest metre.
(Use Mathematical Tabels for this question)

Chapter: [0.05] Trigonometry
Factorize completely using factor theorem:
2x3 – x2 – 13x – 6
Chapter: [0.08] Factorization
Use graph paper to Solution this question.
During a medical checkup of 60 students in a school, weights were recorded as follows:
| Weight (in kg) | Number of Students (f) |
| 28 – 30 | 2 |
| 30 – 32 | 4 |
| 32 – 34 | 10 |
| 34 – 36 | 13 |
| 36 – 38 | 15 |
| 38 – 40 | 9 |
| 40 – 42 | 5 |
| 42 – 44 | 2 |
Taking 2 cm = 2 kg along one axis and 2 cm = 10 students along the other axis draw an ogive. Use your graph to find the:
- median
- upper Quartile
- number of students whose weights is above 37 kg
Chapter: [0.06] Statistics
Other Solutions
Submit Question Paper
Help us maintain new question papers on Shaalaa.com, so we can continue to help studentsonly jpg, png and pdf files
CISCE previous year question papers ICSE Class 10 Mathematics with solutions 2022 - 2023
Previous year Question paper for CISCE ICSE Class 10 Maths-2023 is solved by experts. Solved question papers gives you the chance to check yourself after your mock test.
By referring the question paper Solutions for Mathematics, you can scale your preparation level and work on your weak areas. It will also help the candidates in developing the time-management skills. Practice makes perfect, and there is no better way to practice than to attempt previous year question paper solutions of CISCE ICSE Class 10 .
How CISCE ICSE Class 10 Question Paper solutions Help Students ?
• Question paper solutions for Mathematics will helps students to prepare for exam.
• Question paper with answer will boost students confidence in exam time and also give you an idea About the important questions and topics to be prepared for the board exam.
• For finding solution of question papers no need to refer so multiple sources like textbook or guides.
