Advertisements
Advertisements
प्रश्न
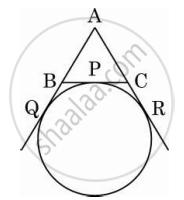
Construct a pair of tangents to a circle of radius 4 cm from a point which is at a distance of 6 cm from its centre.
उत्तर

Step of construction
Step I - First of all, we draw a circle of radius AB = 4 cm.
Step II - Mark a point P from the centre at a distance of 6 cm from the point O.
Step III - Draw a right bisector of OP, intersecting OP at Q.
Step IV - Taking Q as centre and radius OQ = PQ, draw a circle to intersect the given circle at T and T’.
Step V - Join PT and PT’ to obtain the required tangents.
Thus, PT and PT' are the required tangents.
APPEARS IN
संबंधित प्रश्न
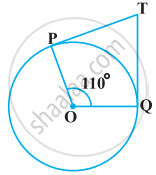
In the given figure, if TP and TQ are the two tangents to a circle with centre O so that ∠POQ = 110°, then ∠PTQ is equal to ______.
Prove that the perpendicular at the point of contact to the tangent to a circle passes through the centre.
The length of a tangent from a point A at distance 5 cm from the centre of the circle is 4 cm. Find the radius of the circle.
Two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
Prove that the tangents drawn at the end points of a chord of a circle make equal angles with the chord.
In the given figure, PQ is a tangent to the circle at A. AB and AD are bisectors of ∠CAQ and ∠PAC. If ∠BAQ = 30°, prove that : BD is diameter of the circle.

Two concentric circles of radii a and b (a > b) are given. Find the length of the chord of the larger circle which touches the smaller circle.
From an external point P, two tangents, PA and PB are drawn to a circle with centre O. At one point E on the circle tangent is drawn which intersects PA and PB at C and D, respectively. If PA = 10 cm, find the the perimeter of the triangle PCD.
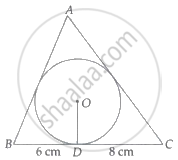
In the given figure, a triangle ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC into which BC is divided by the point of contact D are of lengths 6 cm and 8 cm respectively. If the area of ΔABC is 84 cm2, find the lengths of sides AB and AC.
If a circle is touching the side BC of ΔABC at P and is touching AB and AC produced at Q and R respectively (see the figure). Prove that AQ = `1/2` (perimeter of ΔABC).