Advertisements
Advertisements
प्रश्न
Find the intervals in which the function `f(x) = x^4/4 - x^3 - 5x^2 + 24x + 12` is (a) strictly increasing, (b) strictly decreasing
उत्तर
We have
`f(x) = x^4/4 - x^3 - 5x^2 + 24x + 12`
`=> f'(x) = x^3 -3x^2 - 10x + 24`
As x = 2 satisfies the above equation. Therefore, (x − 2) is a factor.
On performing long division
`(x^3 - 3x^2- 10x + 24)/(x -2) = x^2 -x -12 = (x + 3)(x+4)`
`=> f'(x) = (x - 2) (x + 3) (x -4)`
Here, the critical points are 2, −3, and 4.
The possible intervals are (−∞, −3), (−3, 2), (2, 4), (4, ∞)
a) For f(x) to be strictly increasing, we must have
f'(x) > 0
`=> (x - 2)(x + 3) (x - 4) > 0`
`=> x in (-3,2) U (4,oo)`
So, f(x) is strictly increasing on x∈(−3, 2) ∪ (4, ∞).
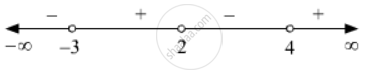
b) For f(x) to be strictly decreasing, we must have
f'(x) < 0
`=> (x - 2)(x+3)(x - 4) < 0`
`=> x in (-oo, -3) U (2,4)``
So, f(x) is strictly decreasing on x∈(−∞, −3) ∪ (2, 4).
APPEARS IN
संबंधित प्रश्न
Show that the function `f(x) = x^3 - 3x^2 + 6x - 100` is increasing on R
Prove that the logarithmic function is strictly increasing on (0, ∞).
Let I be any interval disjoint from (−1, 1). Prove that the function f given by `f(x) = x + 1/x` is strictly increasing on I.
Find the interval in which the following function are increasing or decreasing f(x) = 2x3 − 15x2 + 36x + 1 ?
Find the interval in which the following function are increasing or decreasing f(x) = 2x3 − 24x + 107 ?
Find the interval in which the following function are increasing or decreasing f(x) = x8 + 6x2 ?
Show that the function f given by f(x) = 10x is increasing for all x ?
What are the values of 'a' for which f(x) = ax is increasing on R ?
Write the set of values of 'a' for which f(x) = loga x is decreasing in its domain ?
Write the set of values of a for which the function f(x) = ax + b is decreasing for all x ∈ R ?
Write the interval in which f(x) = sin x + cos x, x ∈ [0, π/2] is increasing ?
The function f(x) = xx decreases on the interval
The function \[f\left( x \right) = \log_e \left( x^3 + \sqrt{x^6 + 1} \right)\] is of the following types:
Function f(x) = 2x3 − 9x2 + 12x + 29 is monotonically decreasing when
f(x) = 2x − tan−1 x − log \[\left\{ x + \sqrt{x^2 + 1} \right\}\] is monotonically increasing when
The function \[f\left( x \right) = \frac{\lambda \sin x + 2 \cos x}{\sin x + \cos x}\] is increasing, if
Let ϕ(x) = f(x) + f(2a − x) and f"(x) > 0 for all x ∈ [0, a]. Then, ϕ (x)
If the function f(x) = x2 − kx + 5 is increasing on [2, 4], then
Find the value of x, such that f(x) is increasing function.
f(x) = x2 + 2x - 5
If the function f(x) = `7/x - 3`, x ∈ R, x ≠ 0 is a decreasing function, then x ∈ ______
The total cost function for production of articles is given as C = 100 + 600x – 3x2, then the values of x for which the total cost is decreasing is ______
The function f(x) = `x - 1/x`, x ∈ R, x ≠ 0 is increasing
The function f(x) = 9 - x5 - x7 is decreasing for
The function f(x) = sin x + 2x is ______
The values of a for which the function f(x) = sinx – ax + b increases on R are ______.
The function f (x) = 2 – 3 x is ____________.
y = log x satisfies for x > 1, the inequality ______.
The intevral in which the function f(x) = 5 + 36x – 3x2 increases will be ______.
Find the interval in which the function f(x) = x2e–x is strictly increasing or decreasing.
