Advertisements
Advertisements
प्रश्न
Find the intervals in which the function `f(x) = x^4/4 - x^3 - 5x^2 + 24x + 12` is (a) strictly increasing, (b) strictly decreasing
Advertisements
उत्तर
We have
`f(x) = x^4/4 - x^3 - 5x^2 + 24x + 12`
`=> f'(x) = x^3 -3x^2 - 10x + 24`
As x = 2 satisfies the above equation. Therefore, (x − 2) is a factor.
On performing long division
`(x^3 - 3x^2- 10x + 24)/(x -2) = x^2 -x -12 = (x + 3)(x+4)`
`=> f'(x) = (x - 2) (x + 3) (x -4)`
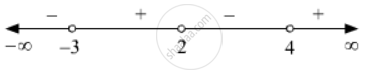
Here, the critical points are 2, −3, and 4.
The possible intervals are (−∞, −3), (−3, 2), (2, 4), (4, ∞)
a) For f(x) to be strictly increasing, we must have
f'(x) > 0
`=> (x - 2)(x + 3) (x - 4) > 0`
`=> x in (-3,2) U (4,oo)`
So, f(x) is strictly increasing on x∈(−3, 2) ∪ (4, ∞).
b) For f(x) to be strictly decreasing, we must have
f'(x) < 0
`=> (x - 2)(x+3)(x - 4) < 0`
`=> x in (-oo, -3) U (2,4)``
So, f(x) is strictly decreasing on x∈(−∞, −3) ∪ (2, 4).
APPEARS IN
संबंधित प्रश्न
Find the value of c in Rolle's theorem for the function `f(x) = x^3 - 3x " in " (-sqrt3, 0)`
Find the intervals in which the function f given by f(x) = 2x3 − 3x2 − 36x + 7 is
- Strictly increasing
- Strictly decreasing
Water is dripping out from a conical funnel of semi-verticle angle `pi/4` at the uniform rate of `2 cm^2/sec`in the surface, through a tiny hole at the vertex of the bottom. When the slant height of the water level is 4 cm, find the rate of decrease of the slant height of the water.
Find the interval in which the following function are increasing or decreasing f(x) = 10 − 6x − 2x2 ?
Find the interval in which the following function are increasing or decreasing f(x) = 2x3 − 12x2 + 18x + 15 ?
Find the interval in which the following function are increasing or decreasing f(x) = x3 − 12x2 + 36x + 17 ?
Show that f(x) = log sin x is increasing on (0, π/2) and decreasing on (π/2, π) ?
Show that the function f(x) = sin (2x + π/4) is decreasing on (3π/8, 5π/8) ?
Find the intervals in which f(x) = log (1 + x) −\[\frac{x}{1 + x}\] is increasing or decreasing ?
Find the set of values of 'b' for which f(x) = b (x + cos x) + 4 is decreasing on R ?
Write the set of values of a for which the function f(x) = ax + b is decreasing for all x ∈ R ?
The function f(x) = xx decreases on the interval
Function f(x) = cos x − 2 λ x is monotonic decreasing when
The function \[f\left( x \right) = \frac{\lambda \sin x + 2 \cos x}{\sin x + \cos x}\] is increasing, if
Find `dy/dx,if e^x+e^y=e^(x-y)`
Find the value of x, such that f(x) is increasing function.
f(x) = 2x3 - 15x2 - 144x - 7
For manufacturing x units, labour cost is 150 – 54x and processing cost is x2. Price of each unit is p = 10800 – 4x2. Find the values of x for which Revenue is increasing.
Find the values of x for which f(x) = 2x3 – 15x2 – 144x – 7 is
- Strictly increasing
- strictly decreasing
A circular pIate is contracting at the uniform rate of 5cm/sec. The rate at which the perimeter is decreasing when the radius of the circle is 10 cm Jong is
For every value of x, the function f(x) = `1/"a"^x`, a > 0 is ______.
The values of k for which the function f(x) = kx3 – 6x2 + 12x + 11 may be increasing on R are ______.
Show that for a ≥ 1, f(x) = `sqrt(3)` sinx – cosx – 2ax + b ∈ is decreasing in R
The function f(x) = x2 – 2x is increasing in the interval ____________.
The function `"f"("x") = "x"/"logx"` increases on the interval
Which of the following graph represent the strictly increasing function.
Let f: [0, 2]→R be a twice differentiable function such that f"(x) > 0, for all x ∈( 0, 2). If `phi` (x) = f(x) + f(2 – x), then `phi` is ______.
If f(x) = x5 – 20x3 + 240x, then f(x) satisfies ______.
Function f(x) = x100 + sinx – 1 is increasing for all x ∈ ______.
