Advertisements
Advertisements
प्रश्न
Find the coordinates of the point Q on the x-axis which lies on the perpendicular bisector of the line segment joining the points A(–5, –2) and B(4, –2). Name the type of triangle formed by the points Q, A and B.
उत्तर
Point Q is the midpoint of AB as the point P lies on the perpendicular bisector of AB.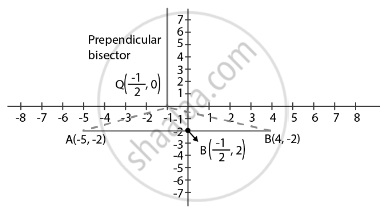
By midpoint formula:
`(x_1 + x_2)/2 = (-5 + 4)/2`
= `-1/2`
x = `-1/2`
Given that,
P lies on x-axis,
So y = 0
P(x, y) = `(-1/2, 0)`
Therefore, it is an isosceles triangle
APPEARS IN
संबंधित प्रश्न
Find equation of line joining (1, 2) and (3, 6) using the determinant.
Find the angle subtended at the origin by the line segment whose end points are (0, 100) and (10, 0).
Show that the following points are collinear:
A(8,1), B(3, -4) and C(2, -5)
In ∆PQR, PR = 8 cm, QR = 4 cm and PL = 5 cm. 
Find:
(i) the area of the ∆PQR
(ii) QM.
If Δ = `|(1, x, x^2),(1, y, y^2),(1, z, z^2)|`, Δ1 = `|(1, 1, 1),(yz, zx, xy),(x, y, z)|`, then prove that ∆ + ∆1 = 0.
Let ∆ = `|("A"x, x^2, 1),("B"y, y^2, 1),("C"z, z^2, 1)|`and ∆1 = `|("A", "B", "C"),(x, y, z),(zy, zx, xy)|`, then ______.
The value of the determinant `abs((1,"x","x"^3),(1,"y","y"^3),(1,"z","z"^3))` is ____________.
The dimensions of a rectangle ABCD are 51 cm × 25 cm. A trapezium PQCD with its parallel sides QC and PD in the ratio 9 : 8, is cut off from the rectangle as shown in the following figure. If the area of the trapezium PQCD is `5/6` th part of the area of the rectangle, find the lengths QC and PD.

Find the missing value:
| Base | Height | Area of parallelogram |
| ______ | 8.4 cm | 48.72 cm2 |
In the given figure, area of ΔPQR is 20 cm2 and area of ΔPQS is 44 cm2. Find the length RS, if PQ is perpendicular to QS and QR is 5 cm.

