Advertisements
Advertisements
प्रश्न
From a point P which is at a distance of 13 cm from the centre O of a circle of radius 5 cm, the pair of tangents PQ and PR to the circle are drawn. Then the area of the quadrilateral PQOR is ______
विकल्प
60 cm2
65 cm2
30 cm2
32.5 cm2
उत्तर
From a point P which is at a distance of 13 cm from the centre O of a circle of radius 5 cm, the pair of tangents PQ and PR to the circle are drawn. Then the area of the quadrilateral PQOR is 60 cm2.
Explanation:
Firstly, draw a circle of radius 5 cm with centre O.
P is a point at a distance of 13 cm from O.
A pair of tangents PQ and PR are drawn.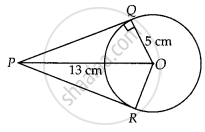
Thus, quadrilateral PQOR is formed.
∵ OQ ⊥ QP ...[Since, QP is a tangent line]
In right angled ∆PQO,
OP2 = OQ2 + QP2
⇒ 132 = 52 + QP2
⇒ QP2 = 169 – 25 = 144
⇒ QP = 12 cm
Now, area of ∆OQP
= `1/2 xx "QP" xx "QO"`
= `1/2 xx 12 xx 5`
= 30 cm2
∴ Area of quadrilateral PQOR
= 2 × ar ∆OQP
= 2 × 30
= 60 cm2
APPEARS IN
संबंधित प्रश्न
In the given figure, O is the centre of the circle. If ∠AOB = 140° and ∠OAC = 50°; find:
- ∠ACB,
- ∠OBC,
- ∠OAB,
- ∠CBA.

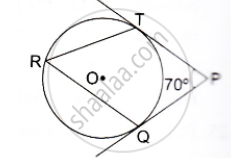
In the given figure, O is the centre of a circle. PT and PQ are tangents to the circle from an external point P. If ∠TPQ = 70° , find the ∠TRQ.
A chord of a circle of radius 14 cm subtends an angle of 120° at the centre. Find the area of the corresponding minor segment of the circle. `[User pi22/7 and sqrt3=1.73]`
In the given figure, ABC is a right triangle right-angled at B such that BC = 6 cm and AB = 8 cm. Find the radius of its incircle.

In the given figure, PO \[\perp\] QO. The tangents to the circle at P and Q intersect at a point T. Prove that PQ and OTare right bisector of each other.

Use the figure given below to fill in the blank:
______ is a chord of the circle.

Can the length of a chord of a circle be greater than its diameter ? Explain.
If a line segment joining mid-points of two chords of a circle passes through the centre of the circle, prove that the two chords are parallel.
From the figure, identify two points in the interior.

From the figure, identify a point in the exterior.

