Advertisements
Advertisements
प्रश्न
If cos θ=0.6 show that (5sin θ -3tan θ) = 0
उत्तर
Let us consider a right ΔABC right angled at B.
Now, we know that cos θ = 0.6 = `(BC)/(AC) = 3/5`
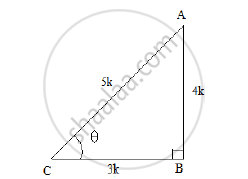
So, if BC = 3k, then AC = 5k, where k is a positive number.
Using Pythagoras theorem, we have:
`Ac^2 = AB^2 + BC^2`
`⟹ AB^2 = AC^2 − BC^2`
`⟹ AB^2 = (5K)^2 − (3K)^2 = 25K^2 − 9K^2`
`⟹ AB^2 = 16K^2`
⟹ 𝐴𝐵 = 4𝑘
Finding out the other T-rations using their definitions, we get:
sin θ = `(AB)/(AC) = (4K)/(5K) = 4/5`
TAN θ = `(AB)/(BC) = (4K)/(3K) = 4/3`
Substituting the values in the given expression, we get:
5 sin 𝜃 − 3 tan 𝜃
`⇒ 5 (4/5) - 3(4/3)`
= 4-4=0= RHS
i.e., LHS = RHS
Hence, Proved.
APPEARS IN
संबंधित प्रश्न
Given: cos A = 0.6; find all other trigonometrical ratios for angle A.
In rectangle ABCD, diagonal BD = 26 cm and cotangent of angle ABD = 1.5. Find the area and the perimeter of the rectangle ABCD.
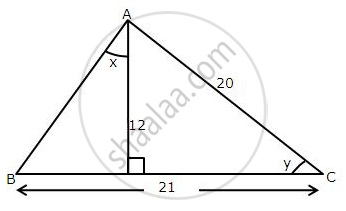
Use the information given in the following figure to evaluate:
`(10)/sin x + (6)/sin y – 6 cot y`.
In ΔABC, ∠A = 90°. If AB = 5 units and AC = 12 units, find: cos C
In ΔABC, ∠B = 90°. If AB = 12units and BC = 5units, find: sinA
In the given figure, ΔABC is right angled at B.AD divides BC in the ratio 1 : 2. Find
(i) `("tan"∠"BAC")/("tan"∠"BAD")` (ii) `("cot"∠"BAC")/("cot"∠"BAD")`
If sin A = `(7)/(25)`, find the value of : cot2A - cosec2A
In the given figure, AC = 13cm, BC = 12 cm and ∠B = 90°. Without using tables, find the values of: `("cos A" - "sin A")/("cos A" + "sin A")`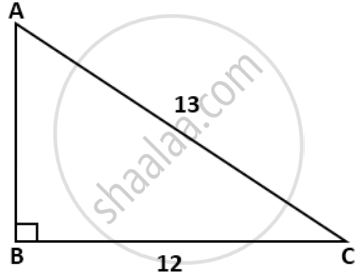
From the given figure, find the values of sec B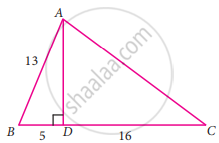
Given that sin α = `1/2` and cos β = `1/2`, then the value of α + β is ______.
