Advertisements
Advertisements
प्रश्न
If the measures of angles of a triangle are in the ratio of 3 : 4 : 5, what is the measure of the smallest angle of the triangle?
विकल्प
25°
30°
45°
60°
उत्तर
In the given figure, measures of the angles of ΔABC are in the ratio 3 : 4 : 5. We need to find the measure of the smallest angle of the triangle.

Let us take,
∠A = 3x
∠B = 4x
∠C = 5x
Now, applying angle sum property of the triangle in ΔABC, we get,
∠A + ∠B + ∠C = 180°
3x + 4x + 5x = 180°
12X = 180°
`x = (180°)/ 12`
x = 15°
Substituting the value of x in ,∠A,∠Band∠C
∠A = 3(15°) = 45
∠B = 4(15V) = 60
∠C = 5(15°) = 75°
Since, the measure of ∠A is the smallest
Thus, the measure of the smallest angle of the triangle is 45°
APPEARS IN
संबंधित प्रश्न
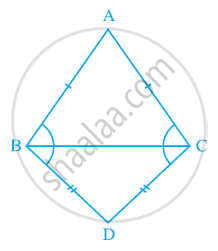
ABC and DBC are two isosceles triangles on the same base BC (see the given figure). Show that ∠ABD = ∠ACD.
The vertical angle of an isosceles triangle is 100°. Find its base angles.
Find the measure of each exterior angle of an equilateral triangle.
Which of the following statements are true (T) and which are false (F):
The measure of each angle of an equilateral triangle is 60°
Which of the following statements are true (T) and which are false (F):
If the bisector of the vertical angle of a triangle bisects the base, then the triangle may be isosceles.
Fill the blank in the following so that the following statement is true.
In right triangles ABC and DEF, if hypotenuse AB = EF and side AC = DE, then ΔABC ≅ Δ ……
In a triangle ABC, if AB = AC and AB is produced to D such that BD = BC, find ∠ACD: ∠ADC.
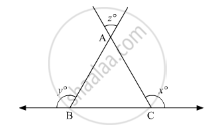
In the given figure, what is z in terms of x and y?
AD is a median of the triangle ABC. Is it true that AB + BC + CA > 2AD? Give reason for your answer.
Show that in a quadrilateral ABCD, AB + BC + CD + DA < 2(BD + AC)
