Advertisements
Advertisements
प्रश्न
If tan x = `3/4` and `pi < x < (3pi)/2`, then find the value of sin `x/2` and cos `x/2`.
उत्तर
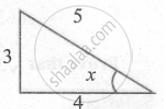
Given tan x = `3/4` and `pi < x < (3pi)/2`
Since x lies in the III quadrant, only tan and its reciprocal are positive.
sin x = `(-3)/5`, cos x = `(-4)/5`.
Now, sin `x/2 = sqrt((1 - cos x)/2) = sqrt(((1 - (-4/5))/2)`
`= sqrt((1 + 4/5)/2)`
`= sqrt(9/10)`
`= 3/sqrt10`
`cos x/2 = sqrt((1 + cos x)/2) = sqrt((1 + 4/5)/2)`
`= sqrt((1 - 4/5)/2) = sqrt(1/10) = 1/sqrt10`
APPEARS IN
संबंधित प्रश्न
Find the value of the following:
cosec 15º
If cot α = `1/2`, sec β = `(-5)/3`, where π < α < `(3pi)/2 and pi/2` < β < π, find the value of tan(α + β). State the quadrant in which α + β terminates.
Prove that:
sin(A + 60°) + sin(A – 60°) = sin A.
If tan θ = 3 find tan 3θ
Prove that cot 4x (sin 5x + sin 3x) = cot x(sin 5x - sin 3x).
Find the value of sin 75°.
If sin A = `1/3`, sin B = `1/4` then find the value of sin (A + B) where A and B are acute angles.
If sin A + cos A = 1 then sin 2A is equal to:
The value of 4 cos3 40° – 3 cos 40° is
If tan A = `1/2` and tan B = `1/3` then tan(2A + B) is equal to:
