Advertisements
Advertisements
प्रश्न
In the following example find the co-ordinate of point A which divides segment PQ in the ratio a : b.
P(–3, 7), Q(1, –4), a : b = 2 : 1
उत्तर
Let the coordinates of point A be (x, y).
P(–3, 7), Q(1, –4), a : b = 2 : 1
Using section formula
\[x = \frac{2 \times 1 + 1 \times \left( - 3 \right)}{2 + 1} = \frac{2 - 3}{3} = \frac{- 1}{3}\]
\[y = \frac{2 \times \left( - 4 \right) + 1 \times 7}{2 + 1} = \frac{- 8 + 7}{3} = \frac{- 1}{3}\]
\[\left( x, y \right) = \left( \frac{- 1}{3}, \frac{- 1}{3} \right)\]
APPEARS IN
संबंधित प्रश्न
Find the mid-point of the line segment joining the points:
(–6, 7) and (3, 5)
Find the mid-point of the line segment joining the points:
(5, –3) and (–1, 7)
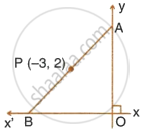
P(–3, 2) is the mid-point of line segment AB as shown in the given figure. Find the co-ordinates of points A and B.
Given a line ABCD in which AB = BC = CD, B = (0, 3) and C = (1, 8). Find the co-ordinates of A and D.
Prove that the points A(–5, 4); B(–1, –2) and C(5, 2) are the vertices of an isosceles right-angled triangle. Find the co-ordinates of D so that ABCD is a square.
Write the co-ordinates of the point of intersection of graphs of
equations x = 2 and y = -3.
Find the midpoint of the line segment joining the following pair of point :
(3a-2b, Sa+7b) and (a+4b, a-3b)
P( -2, 5), Q(3, 6 ), R( -4, 3) and S(-9, 2) are the vertices of a quadrilateral. Find the coordinates of the midpoints of the diagonals PR and QS. Give a special name to the quadrilateral.
If (-3, 2), (1, -2) and (5, 6) are the midpoints of the sides of a triangle, find the coordinates of the vertices of the triangle.
The midpoints of three sides of a triangle are (1, 2), (2, -3) and (3, 4). Find the centroid of the triangle.
Let A(-a, 0), B(0, a) and C(α , β) be the vertices of the L1 ABC and G be its centroid . Prove that
GA2 + GB2 + GC2 = `1/3` (AB2 + BC2 + CA2)
A lies on the x - axis amd B lies on the y -axis . The midpoint of the line segment AB is (4 , -3). Find the coordinates of A and B .
The mid point of the line segment joining the points (p, 2) and (3, 6) is (2, q). Find the numerical values of a and b.
As shown in the figure. two concentric circles are given and line AB is the tangent to the smaller circle at T. Shown that T is the midpoint of Seg AB

The points A(−3, 6), B(0, 7) and C(1, 9) are the mid-points of the sides DE, EF and FD of a triangle DEF. Show that the quadrilateral ABCD is a parallelogram.
The mid-point of the line joining (−a, 2b) and (−3a, −4b) is
In what ratio does the y-axis divides the line joining the points (−5, 1) and (2, 3) internally
The coordinates of diameter AB of a circle are A(2, 7) and B(4, 5), then find the coordinates of the centre
Find coordinates of midpoint of segment joining (– 2, 6) and (8, 2)
Find coordinates of midpoint of the segment joining points (0, 2) and (12, 14)
