Advertisements
Advertisements
प्रश्न
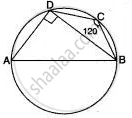
In the adjoining figure, AB is the diameter of the circle with centre O. If ∠BCD = 120°, calculate:
(i) ∠BAD (ii) ∠DBA
उत्तर
(i) Since AOB is a diameter
∴ ∠ADB = 90° ...( C is a semi-circle)
Also, ABCD is a cyclic quadrilateral.
∴ ∠BCD + ∠BAD = 180°
∠BAD = 180° - 120°
⇒ ∠BAD = 60°
(ii) Now, In Δ BAD,
∠BAD + ∠BDA + ∠DBA = 180°
60° + 90° + ∠DBA = 180°
∠DBA = 180° - 150°
∠DBA = 30°
APPEARS IN
संबंधित प्रश्न
PQRS is a cyclic quadrilateral. Given ∠QPS = 73°, ∠PQS = 55° and ∠PSR = 82°, calculate:
1) ∠QRS
2) ∠RQS
3) ∠PRQ

In cyclic quadrilateral ABCD, ∠A = 3∠C and ∠D = 5∠B. Find the measure of each angle of the quadrilateral.
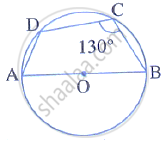
In the given figure, AB is the diameter of a circle with centre O. ∠BCD = 130°. Find:
(i) ∠DAB
(ii) ∠DBA
In a cyclic-quadrilateral PQRS, angle PQR = 135°. Sides SP and RQ produced meet at point A whereas sides PQ and SR produced meet at point B. If ∠A : ∠B = 2 : 1; find angles A and B.
In following figure , Δ PQR is an isosceles teiangle with PQ = PR and m ∠ PQR = 35° .Find m ∠ QSR and ∠ QTR

In following fig., O is the centre of the circle. Find ∠ CBD.

In the following figure, O is the centre of the circle. Find the values of a, b and c.

In the figure, given below, find: ∠ABC. Show steps of your working.

In the figure, O is the centre of the circle and the length of arc AB is twice the length of arc BC. If angle AOB = 108°, find: ∠ADB.
If ABCD is a cyclic quadrilateral in which AD || BC. Prove that ∠B = ∠C.