Advertisements
Advertisements
प्रश्न
PQRS is a cyclic quadrilateral. Given ∠QPS = 73°, ∠PQS = 55° and ∠PSR = 82°, calculate:
1) ∠QRS
2) ∠RQS
3) ∠PRQ

उत्तर
Given: PQRS is a cyclic quadrilateral.
∠QPS = 73°, ∠PQS = 55° and ∠PSR = 82°
1) Opposite angle of a cyclic quadrilateral is supplementary.
⇒ ∠QPS + ∠QRS = 180°
⇒ 73° + ∠QRS = 180°
⇒ ∠QRS = 180° - 73= 107°
2) Opposite angle of a cyclic quadrilateral are supplementary.
⇒ ∠PSR + ∠PQR = 180°
⇒ ∠PSR + (⇒ ∠PQS + ⇒ ∠RQS) = 180°
⇒ 82° + 55° + ∠RQS = 180°
⇒ ∠RQS = 180° - 137° = 43°
3) In ΔPQS, by angle sum property, we have
⇒ ∠PSQ + ∠PQS + ∠QPS = 180°
⇒ ∠PSQ + 55° + 73° = 180°
⇒ ∠PSQ = 180° - 128° = 52°
Now ⇒ ∠PRQ = ∠PSQ (angles in the same segment of a circle)
⇒ ∠PRQ = 52°
APPEARS IN
संबंधित प्रश्न
In the figure, given below, find:
- ∠BCD,
- ∠ADC,
- ∠ABC.
Show steps of your working.

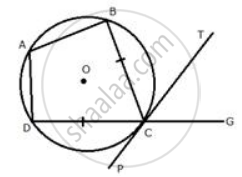
In the figure, ABCD is a cyclic quadrilateral with BC = CD. TC is tangent to the circle at point C and DC is produced to point G. If ∠BCG = 108° and O is the centre of the circle, find :
- angle BCT
- angle DOC

In cyclic quadrilateral ABCD, ∠A = 3∠C and ∠D = 5∠B. Find the measure of each angle of the quadrilateral.
The quadrilateral formed by angle bisectors of a cyclic quadrilateral is also cyclic. Prove it.
ABCD is a cyclic quadrilateral of a circle with centre O such that AB is a diameter of this circle and the length of the chord CD is equal to the radius of the circle. If AD and BC produced meet at P, show that APB = 60°.
In a cyclic quadrilateral ABCD , AB || CD and ∠ B = 65 ° , find the remaining angles

In the figure, ABCD is a cyclic quadrilateral with BC = CD. TC is tangent to the circle at point C and DC is produced to point G. If angle BCG=108° and O is the centre of the circle, find: angle DOC
In the given figure, AB is the diameter. The tangent at C meets AB produced at Q.

If ∠ CAB = 34° , find : ∠ CQB
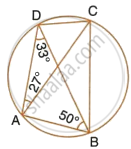
In cyclic quadrilateral ABCD, ∠DAC = 27°; ∠DBA = 50° and ∠ADB = 33°. Calculate : ∠DCB.
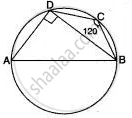
In the adjoining figure, AB is the diameter of the circle with centre O. If ∠BCD = 120°, calculate:
(i) ∠BAD (ii) ∠DBA