Advertisements
Advertisements
Question
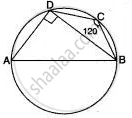
In the adjoining figure, AB is the diameter of the circle with centre O. If ∠BCD = 120°, calculate:
(i) ∠BAD (ii) ∠DBA
Solution
(i) Since AOB is a diameter
∴ ∠ADB = 90° ...( C is a semi-circle)
Also, ABCD is a cyclic quadrilateral.
∴ ∠BCD + ∠BAD = 180°
∠BAD = 180° - 120°
⇒ ∠BAD = 60°
(ii) Now, In Δ BAD,
∠BAD + ∠BDA + ∠DBA = 180°
60° + 90° + ∠DBA = 180°
∠DBA = 180° - 150°
∠DBA = 30°
APPEARS IN
RELATED QUESTIONS
In the figure, given below, find:
- ∠BCD,
- ∠ADC,
- ∠ABC.
Show steps of your working.

In the following figure,
- if ∠BAD = 96°, find ∠BCD and ∠BFE.
- Prove that AD is parallel to FE.

ABCD is a cyclic quadrilateral in which BC is parallel to AD, angle ADC = 110° and angle BAC = 50°. Find angle DAC and angle DCA.
The quadrilateral formed by angle bisectors of a cyclic quadrilateral is also cyclic. Prove it.
In the following figure, O is the centre of the circle. Find the values of a, b and c.

In the following figure, Prove that AD is parallel to FE.

In the given below figure,
∠ BAD = 65°
∠ ABD = 70°
∠ BDC = 45°
Find: (i) ∠ BCD, (ii) ∠ ADB.
Hence show that AC is a diameter.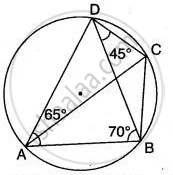
If O is the centre of the circle, find the value of x in each of the following figures

In the given circle with centre O, ∠ABC = 100°, ∠ACD = 40° and CT is a tangent to the circle at C. Find ∠ADC and ∠DCT.

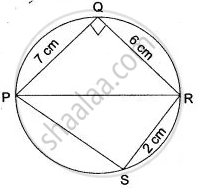
In the figure alongside PR is a diameter of the circle, PQ = 7 cm; QR = 6 cm and RS = 2 cm. Calculate the perimeter of the cyclic quadrilateral PQRS.