Advertisements
Advertisements
प्रश्न
In the figure below, find the value of 'x'.

उत्तर
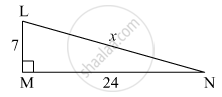
In the right-angled triangle LMN,
∠M = 90°
∴ Side LN is the hypotenuse.
According to Pythagoras' theorem,
l(LN)2 = l(LM)2 + l(MN)2
⇒ (x)2 = (7)2 + (24)2
⇒ x2 = 49 + 576
⇒ x2 = 625
⇒ x = `root 625`
⇒ x = 25
∴ The value of x is 25.
संबंधित प्रश्न
If ABC is an equilateral triangle of side a, prove that its altitude = ` \frac { \sqrt { 3 } }{ 2 } a`
PQR is a triangle right angled at P and M is a point on QR such that PM ⊥ QR. Show that PM2 = QM . MR
In Figure ABD is a triangle right angled at A and AC ⊥ BD. Show that AC2 = BC × DC

Prove that the sum of the squares of the sides of a rhombus is equal to the sum of the squares of its diagonals
Pranali and Prasad started walking to the East and to the North respectively, from the same point and at the same speed. After 2 hours distance between them was \[15\sqrt{2}\]
km. Find their speed per hour.
Triangle PQR is right-angled at vertex R. Calculate the length of PR, if: PQ = 34 cm and QR = 33.6 cm.
A ladder 15m long reaches a window which is 9m above the ground on one side of a street. Keeping its foot at the same point, the ladder is turned to other side of the street to reach a window 12m high. Find the width of the street.
In a triangle ABC right angled at C, P and Q are points of sides CA and CB respectively, which divide these sides the ratio 2 : 1.
Prove that: 9BP2 = 9BC2 + 4AC2
Rithika buys an LED TV which has a 25 inches screen. If its height is 7 inches, how wide is the screen? Her TV cabinet is 20 inches wide. Will the TV fit into the cabinet? Give reason
Points A and B are on the opposite edges of a pond as shown in figure. To find the distance between the two points, the surveyor makes a right-angled triangle as shown. Find the distance AB.

