Advertisements
Advertisements
प्रश्न
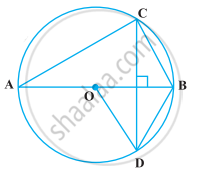
In the following figure, O is the centre of the circle, BD = OD and CD ⊥ AB. Find ∠CAB.
Advertisements
उत्तर
Given, in the figure BD = OD, CD ⊥ AB.
In ΔOBD, BD = OD ...[Given]
OD = OB ...[Both are the radius of circle]
∴ OB = OD = BD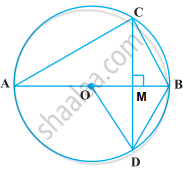
Thus, ΔODB is an equilateral triangle.
∴ ∠BOD = ∠OBD = ∠ODB = 60°
In ΔMBC and ΔMBD,
MB = MB ...[Common side]
∠CMB = ∠BMD = 90°
And CM = MD ...[In a circle, any perpendicular drawn on a chord also bisects the chord]
∴ ΔMBC ≅ ΔMBD ...[By SAS congruence rule]
∴ ∠MBC = ∠MBD ...[By CPCT]
⇒ ∠MBC = ∠OBD = 60° ...[∵ ∠OBD = 60°]
Since, AB is a diameter of the circle.
∴ ∠ACB = 90°
In ΔACB, ∠CAB + ∠CBA + ∠ACB = 180° ...[By angle sum property of a triangle]
⇒ ∠CAB + 60° + 90° = 180°
⇒ ∠CAB = 180° – (60° + 90°) = 30°
APPEARS IN
संबंधित प्रश्न
In the given figure, the incircle of ∆ABC touches the sides BC, CA and AB at D, E, F respectively. Prove that AF + BD + CE = AE + CD + BF = `\frac { 1 }{ 2 } ("perimeter of ∆ABC")`
Fill in the blank
A continuous piece of a circle is ............... of the circle
In the given figure, two tangents RQ and RP are drawn from an external point R to the circle with centre O. If ∠PRQ = 120°, then prove that OR = PR + RQ.
The perimeter (in cm) of a square circumscribing a circle of radius a cm, is
In the given figure, O is the centre of the circle. Find ∠CBD.
In the given figure, if ABC is an equilateral triangle. Find ∠BDC and ∠BEC.
The length of three concesutive sides of a quadrilateral circumscribing a circle are 4 cm, 5 cm, and 7 cm respectively. Determine the length of the fourth side.
Use the figure given below to fill in the blank:
Diameter = 2 x ________
Use the figure given below to fill in the blank:
AB is a ______ of the circle.
Circles with centres A, B and C touch each other externally. If AB = 3 cm, BC = 3 cm, CA = 4 cm, then find the radii of each circle.
