Advertisements
Advertisements
प्रश्न
In triangle ABC, AB = AC. A circle passing through B and c intersects the sides AB and AC at D and E respectively. Prove that DE || BC.
उत्तर १
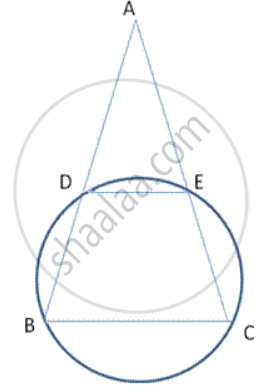
To prove = DE II BC
Proof: In cydic quadrilateral DECB
∠ DEC + ∠ DBC = 80° - ( 1) (Opposite angles of cyclic quadrilateral)
Also, ∠ AED + ∠ DEC = 80° - (2) (Linear pair)
From (1) and (2), we get,
∠ DBC = ∠ AED - (3)
AB= AC (given)
∴ ∠ABC = ∠ ACB - (4) (angles opposite to equal sides of triangle)
From (3) and ( 4) ⇒ ∠ AED = ∠ ACB
But, these are corresponding angles
∴ DE II BC
उत्तर २
In order to prove that DE || BC, it is sufficient to show that ∠B = ∠ADE.
In Δ ABC, we have
AB = AC ⇒ ∠B = ∠C ...(i)
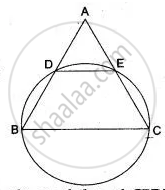
In the cyclic quadrilateral CBDE, side BD is produced to A.
∴ ∠ADE = ∠C ...(ii)( ∵ Exterior angle = Opposite interior angle )
From (i) and (ii), we get
∠B = ∠ADE.
Hence, DE || BC. ...Hence proved.
APPEARS IN
संबंधित प्रश्न
ABCD is a quadrilateral inscribed in a circle, having ∠ = 60°; O is the center of the circle.
Show that: ∠OBD + ∠ODB =∠CBD +∠CDB.
ABCD is a cyclic quadrilateral. Sides AB and DC produced meet at point E; whereas sides BC and AD produced meet at point F. If ∠DCF : ∠F : ∠E = 3 : 5 : 4, find the angles of the cyclic quadrilateral ABCD.
Two circles intersect in points P and Q. A secant passing through P intersects the circles in A and B respectively. Tangents to the circles at A and B intersect at T. Prove that A, Q, B and T lie on a circle.
Prove that any four vertices of a regular pentagon are concylic (lie on the same circle).
In the given figure, AB is parallel to DC, ∠BCE = 80° and ∠BAC = 25°.

Find:
- ∠CAD
- ∠CBD
- ∠ADC
In the figure, ∠DBC = 58°. BD is a diameter of the circle. Calculate : ∠BAC

ABCD is a cyclic quadrilateral AB and DC are produced to meet in E. Prove that Δ EBC ∼ Δ EDA.
Prove that the angle bisectors of the angles formed by producing opposite sides of a cyclic quadrilateral (Provided they are not parallel) intersect at the right angle.
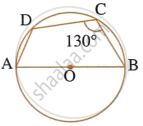
In the given figure, AB is the diameter of a circle with centre O.
∠BCD = 130°. Find:
- ∠DAB
- ∠DBA
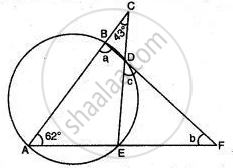
In the given figure, if ∠ ACE = 43° and ∠CAF = 62°. Find the value of a, b, and c.