Advertisements
Advertisements
प्रश्न
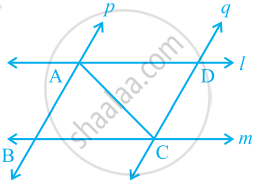
l and m are two parallel lines intersected by another pair of parallel lines p and q (see the given figure). Show that ΔABC ≅ ΔCDA.
उत्तर
l || m ...[Given]
AC is a transversal.
So, ∠DAC = ∠ACB ...[Alternate angles]
p || q ...[Given]
AC is a transversal.
So, ∠BAC = ∠ACD ...[Alternate angles]
Now, △ABC and △CDA,
∠ACB = ∠DAC ...[Proved above]
∠BAC = ∠ACD ...[Proved above]
AC = AC ...[Common]
△ABC ≌ △CDA ...[By AAS congruence rule]
APPEARS IN
संबंधित प्रश्न
You want to show that ΔART ≅ ΔPEN,
If you have to use SSS criterion, then you need to show
1) AR =
2) RT =
3) AT =

If ABC and DEF are two triangles such that AC = 2.5 cm, BC = 5 cm, ∠C = 75°, DE = 2.5 cm, DF = 5cm and ∠D = 75°. Are two triangles congruent?
In two triangles ABC and ADC, if AB = AD and BC = CD. Are they congruent?
In a triangle ABC, D is mid-point of BC; AD is produced up to E so that DE = AD. Prove that:
AB is parallel to EC.
In ∆ABC, AB = AC. Show that the altitude AD is median also.
In the following figure, BL = CM.

Prove that AD is a median of triangle ABC.
In the adjoining figure, QX and RX are the bisectors of the angles Q and R respectively of the triangle PQR.
If XS ⊥ QR and XT ⊥ PQ ;
prove that: (i) ΔXTQ ≅ ΔXSQ.
(ii) PX bisects angle P.
A point O is taken inside a rhombus ABCD such that its distance from the vertices B and D are equal. Show that AOC is a straight line.
In the following figure, ∠A = ∠C and AB = BC.
Prove that ΔABD ≅ ΔCBE. 
PQRS is a parallelogram. L and M are points on PQ and SR respectively such that PL = MR.
Show that LM and QS bisect each other.
