Advertisements
Advertisements
प्रश्न
Let f : R `{- 4/3} `- 43 →">→ R be a function defined as f(x) = `(4x)/(3x +4)` . Show that f : R - `{-4/3}`→ Rang (f) is one-one and onto. Hence, find f -1.
उत्तर
The function f : R - `{-4/3}→ R - {4/3}` is given by f (x) = `(4x)/(3x +4).` Injectivity: Let `x,y in R - {-4/3} `be such that f (x) = f (y)
⇒ `(4x)/(3x + 4)= (4x)/(3y+4)`
⇒ 4x (3y +4) = 4y (3x +4)
⇒ 12xy + 16x = 12xy +16y
⇒ 16x = 16y
⇒ x = y
Hence, f is one-one function.
Surjectivity: Let y be an arbitrary element of `R - {4/3}.` Then,
f(x) = y
⇒ `(4x)/(3x+4) = y`
⇒ 4x = 3xy +4y
⇒ 4x - 3xy = 4y
⇒ `x = (4y)/(4-3y)`
As `y ∈ R -{4/3} , (4y)/(4-3y) in R.`
Also , `(4y)/(4 - 3y) ≠ -4/3` because `(4y)/(4-3y) = - 4/3 ⇒ 12y = -16 +12y ⇒ 0 = -16,`which is not posssible.
Thus,
`x = (4y)/(4-3y) in R - {- 4/3}` sich that
`f (x) = f ((4x)/(3x +4))= (4((4y)/(4 -3y)))/(3((4y)/(4 -3y))+4) = (16y)/(12y+ 16 - 12y)= (16y)/16` = y , so every element in `R - {4/3} ` has pre-image in `R- {-4/3}.`
Hence, f is onto.
Now,
`x = (4y)/(4 -3y)`
Replacing x by f-1 and y by x, we have
`f^-1 (x) = (4x)/(4 - 3x)`
APPEARS IN
संबंधित प्रश्न
Show that function f: R `rightarrow` {x ∈ R : −1 < x < 1} defined by f(x) = `x/(1 + |x|)`, x ∈ R is one-one and onto function.
Let f: R → R be the Signum Function defined as
f(x) = `{(1,x>0), (0, x =0),(-1, x< 0):}`
and g: R → R be the Greatest Integer Function given by g(x) = [x], where [x] is greatest integer less than or equal to x. Then does fog and gof coincide in (0, 1]?
Classify the following function as injection, surjection or bijection :
f : Z → Z, defined by f(x) = x − 5
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = x3 + 1
Find gof and fog when f : R → R and g : R → R is defined by f(x) = 8x3 and g(x) = x1/3.
Verify associativity for the following three mappings : f : N → Z0 (the set of non-zero integers), g : Z0 → Q and h : Q → R given by f(x) = 2x, g(x) = 1/x and h(x) = ex.
Let f be any real function and let g be a function given by g(x) = 2x. Prove that gof = f + f.
Let
f (x) =`{ (1 + x, 0≤ x ≤ 2) , (3 -x , 2 < x ≤ 3):}`
Find fof.
Consider f : {1, 2, 3} → {a, b, c} and g : {a, b, c} → {apple, ball, cat} defined as f (1) = a, f (2) = b, f (3) = c, g (a) = apple, g (b) = ball and g (c) = cat. Show that f, g and gof are invertible. Find f−1, g−1 and gof−1and show that (gof)−1 = f −1o g−1
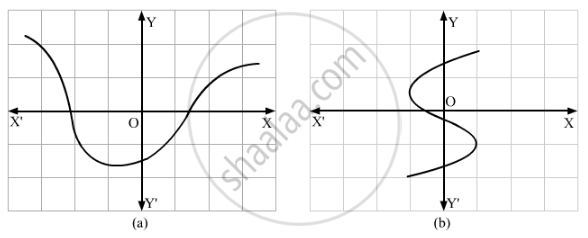
Which one of the following graphs represents a function?
If A = {1, 2, 3} and B = {a, b}, write the total number of functions from A to B.
If A = {a, b, c} and B = {−2, −1, 0, 1, 2}, write the total number of one-one functions from A to B.
Let f : R − {−1} → R − {1} be given by\[f\left( x \right) = \frac{x}{x + 1} . \text{Write } f^{- 1} \left( x \right)\]
What is the range of the function
`f (x) = ([x - 1])/(x -1) ?`
If f(x) = 4 −( x - 7)3 then write f-1 (x).
The range of the function
\[f\left( x \right) =^{7 - x} P_{x - 3}\]
Let
The function \[f : R \to R\] defined by
\[f\left( x \right) = 6^x + 6^{|x|}\] is
If \[F : [1, \infty ) \to [2, \infty )\] is given by
\[f\left( x \right) = x + \frac{1}{x}, then f^{- 1} \left( x \right)\]
Let A = ℝ − {3}, B = ℝ − {1}. Let f : A → B be defined by \[f\left( x \right) = \frac{x - 2}{x - 3}, \forall x \in A\] Show that f is bijective. Also, find
(i) x, if f−1(x) = 4
(ii) f−1(7)
If f(x) = `(x+3)/(4x−5) , "g"(x) = (3+5x)/(4x−1)` then verify that `("fog") (x)` = x.
Which function is used to check whether a character is alphanumeric or not?
Let the function f: R → R be defined by f(x) = cosx, ∀ x ∈ R. Show that f is neither one-one nor onto
Let X = {1, 2, 3}and Y = {4, 5}. Find whether the following subset of X ×Y are function from X to Y or not
f = {(1, 4), (1, 5), (2, 4), (3, 5)}
Let A = R – {3}, B = R – {1}. Let f: A → B be defined by f(x) = `(x - 2)/(x - 3)` ∀ x ∈ A . Then show that f is bijective
Let A = [–1, 1]. Then, discuss whether the following functions defined on A are one-one, onto or bijective:
g(x) = |x|
Let A = [–1, 1]. Then, discuss whether the following functions defined on A are one-one, onto or bijective:
h(x) = x|x|
If the set A contains 5 elements and the set B contains 6 elements, then the number of one-one and onto mappings from A to B is ______.
Let f: `[2, oo)` → R be the function defined by f(x) = x2 – 4x + 5, then the range of f is ______.
The function f: R → R defined as f(x) = x3 is:
Sherlin and Danju are playing Ludo at home during Covid-19. While rolling the dice, Sherlin’s sister Raji observed and noted the possible outcomes of the throw every time belongs to set {1,2,3,4,5,6}. Let A be the set of players while B be the set of all possible outcomes.
A = {S, D}, B = {1,2,3,4,5,6}
- Raji wants to know the number of functions from A to B. How many number of functions are possible?
An organization conducted a bike race under 2 different categories-boys and girls. Totally there were 250 participants. Among all of them finally, three from Category 1 and two from Category 2 were selected for the final race. Ravi forms two sets B and G with these participants for his college project. Let B = {b1,b2,b3} G={g1,g2} where B represents the set of boys selected and G the set of girls who were selected for the final race.
Ravi decides to explore these sets for various types of relations and functions.
- Let R: B → G be defined by R = { (b1,g1), (b2,g2),(b3,g1)}, then R is ____________.
An organization conducted a bike race under 2 different categories-boys and girls. Totally there were 250 participants. Among all of them finally, three from Category 1 and two from Category 2 were selected for the final race. Ravi forms two sets B and G with these participants for his college project. Let B = {b1,b2,b3} G={g1,g2} where B represents the set of boys selected and G the set of girls who were selected for the final race.
Ravi decides to explore these sets for various types of relations and functions.
- Ravi wants to find the number of injective functions from B to G. How many numbers of injective functions are possible?
Let f: R → R defined by f(x) = 3x. Choose the correct answer
Let x is a real number such that are functions involved are well defined then the value of `lim_(t→0)[max{(sin^-1 x/3 + cos^-1 x/3)^2, min(x^2 + 4x + 7)}]((sin^-1t)/t)` where [.] is greatest integer function and all other brackets are usual brackets.
If f: [0, 1]→[0, 1] is defined by f(x) = `(x + 1)/4` and `d/(dx) underbrace(((fofof......of)(x)))_("n" "times")""|_(x = 1/2) = 1/"m"^"n"`, m ∈ N, then the value of 'm' is ______.
Let f(x) be a polynomial of degree 3 such that f(k) = `-2/k` for k = 2, 3, 4, 5. Then the value of 52 – 10f(10) is equal to ______.
Find the domain of sin–1 (x2 – 4).
