Advertisements
Advertisements
प्रश्न
Let f: R → R be defined as f(x) = x4. Choose the correct answer.
विकल्प
f is one - one onto
f is many - one onto
f is one - one but not onto
f is neither one - one nor onto
उत्तर
f is neither one - one nor onto
Explanation:
f: R → R is defined as `f(x) = x^4`
Let x, y ∈ R such that f(x) = f(y).
` =>x^4 = y^4`
`=> x=+-y`
∴`f(x_1) = f(x_2)` does not imply that `x_1 = x_2`
For instance,
f(1) = f(-1) = 1
∴ f is not one-one.
Consider an element 2 in co-domain R. It is clear that there does not exist any x in domain R such that f(x) = 2.
∴ f is not onto.
Hence, function f is neither one-one nor onto.
The correct answer is D.
APPEARS IN
संबंधित प्रश्न
Show that the function f: R* → R* defined by `f(x) = 1/x` is one-one and onto, where R* is the set of all non-zero real numbers. Is the result true if the domain R* is replaced by N, with co-domain being same as R?
Let f: R → R be defined as f(x) = 10x + 7. Find the function g: R → R such that g o f = f o g = 1R.
Show that the function f: R → R given by f(x) = x3 is injective.
Let S = {a, b, c} and T = {1, 2, 3}. Find F−1 of the following functions F from S to T, if it exists.
F = {(a, 3), (b, 2), (c, 1)}
Let A = {−1, 0, 1, 2}, B = {−4, −2, 0, 2} and f, g: A → B be functions defined by f(x) = x2 − x, x ∈ A and g(x) = `2|x - 1/2|- 1, x in A`. Are f and g equal?
Justify your answer. (Hint: One may note that two functions f: A → B and g: A → B such that f(a) = g(a) ∀ a ∈ A are called equal functions).
Classify the following function as injection, surjection or bijection : f : N → N given by f(x) = x3
Classify the following function as injection, surjection or bijection : f : Z → Z given by f(x) = x3
Classify the following function as injection, surjection or bijection :
f : R → R, defined by f(x) = 3 − 4x
If f : A → B is an injection, such that range of f = {a}, determine the number of elements in A.
Find gof and fog when f : R → R and g : R → R is defined by f(x) = 2x + x2 and g(x) = x3
Let f : R → R and g : R → R be defined by f(x) = x + 1 and g (x) = x − 1. Show that fog = gof = IR.
Consider f : N → N, g : N → N and h : N → R defined as f(x) = 2x, g(y) = 3y + 4 and h(z) = sin z for all x, y, z ∈ N. Show that ho (gof) = (hog) of.
Find fog and gof if : f (x) = |x|, g (x) = sin x .
Find f −1 if it exists : f : A → B, where A = {1, 3, 5, 7, 9}; B = {0, 1, 9, 25, 49, 81} and f(x) = x2
Consider f : {1, 2, 3} → {a, b, c} and g : {a, b, c} → {apple, ball, cat} defined as f (1) = a, f (2) = b, f (3) = c, g (a) = apple, g (b) = ball and g (c) = cat. Show that f, g and gof are invertible. Find f−1, g−1 and gof−1and show that (gof)−1 = f −1o g−1
If f : R → (0, 2) defined by `f (x) =(e^x - e^(x))/(e^x +e^(-x))+1`is invertible , find f-1.
Let A and B be two sets, each with a finite number of elements. Assume that there is an injective map from A to B and that there is an injective map from B to A. Prove that there is a bijection from A to B.
Write the domain of the real function
`f (x) = sqrt([x] - x) .`
What is the range of the function
`f (x) = ([x - 1])/(x -1) ?`
\[f : A \to \text{B given by } 3^{ f\left( x \right)} + 2^{- x} = 4\] is a bijection, then
Let
\[A = \left\{ x \in R : - 1 \leq x \leq 1 \right\} = B\] Then, the mapping\[f : A \to \text{B given by} f\left( x \right) = x\left| x \right|\] is
Let
Mark the correct alternative in the following question:
Let A = {1, 2, ... , n} and B = {a, b}. Then the number of subjections from A into B is
Set A has 3 elements and the set B has 4 elements. Then the number of injective mappings that can be defined from A to B is ______.
Let f: R → R be defined by f(x) = 3x – 4. Then f–1(x) is given by ______.
Let f: R → R be the function defined by f(x) = 2x – 3 ∀ x ∈ R. write f–1
The number of bijective functions from set A to itself when A contains 106 elements is ____________.
Let f : R `->` R be a function defined by f(x) = x3 + 4, then f is ______.
The function f: R → R defined as f(x) = x3 is:
Given a function If as f(x) = 5x + 4, x ∈ R. If g : R → R is inverse of function ‘f then
If f: R → R given by f(x) =(3 − x3)1/3, find f0f(x)
A function f: x → y is said to be one – one (or injective) if:
Let f: R→R be a continuous function such that f(x) + f(x + 1) = 2, for all x ∈ R. If I1 = `int_0^8f(x)dx` and I2 = `int_(-1)^3f(x)dx`, then the value of I1 + 2I2 is equal to ______.
`x^(log_5x) > 5` implies ______.
The domain of function is f(x) = `sqrt(-log_0.3(x - 1))/sqrt(x^2 + 2x + 8)` is ______.
For x ∈ R, x ≠ 0, let f0(x) = `1/(1 - x)` and fn+1 (x) = f0(fn(x)), n = 0, 1, 2, .... Then the value of `f_100(3) + f_1(2/3) + f_2(3/2)` is equal to ______.
Which one of the following graphs is a function of x?
 |
 |
| Graph A | Graph B |
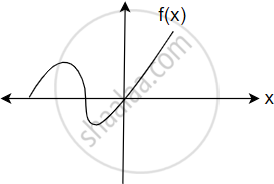
The given function f : R → R is not ‘onto’ function. Give reason.
