Advertisements
Advertisements
प्रश्न
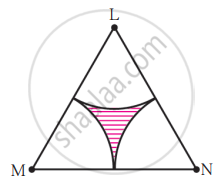
(2) Area of any one of the sectors
उत्तर
Given,
ΔLMN is an equilateral triangle.
LM = 14 cm
Radius of the sectors (r) = 7 cm
(1) ΔLMN is an equilateral triangle. LM = 14 cm ...(Given)
Area of an equilateral triangle is `sqrt3/4 ("side")^2`.
A(ΔLMN) = `sqrt3/4 (14)^2`
A(ΔLMN) = `sqrt3/4 × 196`
A(ΔLMN) = `sqrt3 × 49`
A(ΔLMN) = 1.732 × 49
A(ΔLMN) = 84.868 ≈ 84.87 cm2
∴ The area of ΔLMN is 84.87 cm2.
(2) We know that, all the angles of the equilateral triangle are equal. Thus, ∠L = ∠M = ∠N = 60°.
Central angle (θ) = 60°
Area of sector = `θ/360 × πr^2`
= `60/360 × 22/7 × 7^2`
= `1/6 × 22 × 7`
= `(11 × 7)/3`
= `77/3`
= 25.67 cm2
∴ Area of one sector = 25.67 cm2
(3) Total area of all the three sectors = 3 × Area of one sector
= 3 × 25.67
= 77.01 cm2
(4) Area of shaded region = A(∆LMN) – total area of all three sectors
= 84.87 − 77.01
= 7.86 cm2
∴ Area of shaded region = 7.86 cm2.
(2) Area of any one of the sectors = 25.67 cm2
APPEARS IN
संबंधित प्रश्न
Find the area of the sector whose arc length and radius are 14 cm and 6 cm respectively.
The length of the minute hand of a clock is 14 cm. Find the area swept by the minute hand in 5 minutes. [Use `pi = 22/7`]
A chord of a circle of radius 15 cm subtends an angle of 60° at the centre. Find the areas of the corresponding minor and major segments of the circle. [Use π = 3.14 and `sqrt3 = 1.73`]
A sector of circle of radius 4cm contains an angle of 30°. Find the area of sector
The area of sector of circle of radius 2cm is 𝜋cm2. Find the angle contained by the sector.
AB is a chord of a circle with centre O and radius 4 cm. AB is of length 4 cm and divides the circle into two segments. Find the area of the minor segment.
A chord of circle of radius 14cm makes a right angle at the centre. Find the areas of minor and major segments of the circle.

In the given figure, if A is the centre of the circle. \[\angle\] PAR = 30°, AP = 7.5, find the area of the segment PQR. (\[\pi\] = 3.14)

The length of the minute hand of a clock is 5 cm. Find the area swept by the minute hand during the time period 6 : 05 am and 6 : 40 am.
In the given figure, three sectors of a circle of radius 7 cm, making angles of 60°, 80° and 40° at the centre are shaded. Find the area of the shaded region.

In a circle of radius 7 cm, a square ABCD is inscribed. Find the area of the circle which is outside the square.
A chord of a circle of radius 10 cm subtends a right angle at the centre. Find the area of the minor segment. [Use π = 3.14.]
In following fig., PT is a tangent to the circle at T and PAB is a secant to the same circle. If PA = 4cm and AB = Scm, find PT.
The area of the largest square that can be inscribed in a circle of radius 12 cm is ____________.
If angle of sector is 60°, radius is 3.5 cm then length of the arc is ____________.
Sides of a triangular field are 15 m, 16 m and 17 m. With the three corners of the field a cow, a buffalo and a horse are tied separately with ropes of length 7 m each to graze in the field. Find the area of the field which cannot be grazed by the three animals.
What is the length of arc of a circle of radius 7 cm which subtends an angle of 90° at the centre of the circle?
The area of the sector of a circle of radius 12 cm is 60π cm2. The central angle of this sector is ______.
In a circle of radius 21 cm, an arc subtends an angle of 60° at the centre. Find the area of the segment formed by the corresponding chord. (Use π = `22/7`)
