Advertisements
Advertisements
प्रश्न
Prove that the sum of the squares of the diagonals of parallelogram is equal to the sum of the squares of its sides.
उत्तर
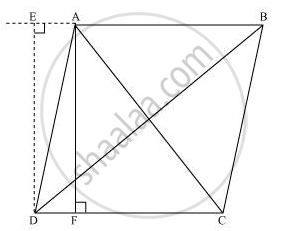
Let ABCD be a parallelogram.
Let us draw perpendicular DE on extended side AB, and AF on side DC.
Applying Pythagoras theorem in ΔDEA, we obtain
DE2 + EA2 = DA2 … (i)
Applying Pythagoras theorem in ΔDEB, we obtain
DE2 + EB2 = DB2
DE2 + (EA + AB)2 = DB2
(DE2 + EA2) + AB2 + 2EA × AB = DB2
DA2 + AB2 + 2EA × AB = DB2 … (ii)
Applying Pythagoras theorem in ΔADF, we obtain
AD2 = AF2 + FD2
Applying Pythagoras theorem in ΔAFC, we obtain
AC2 = AF2 + FC2
= AF2 + (DC − FD)2
= AF2 + DC2 + FD2 − 2DC × FD
= (AF2 + FD2) + DC2 − 2DC × FD
AC2 = AD2 + DC2 − 2DC × FD … (iii)
Since ABCD is a parallelogram,
AB = CD … (iv)
And, BC = AD … (v)
In ΔDEA and ΔADF,
∠DEA = ∠AFD (Both 90°)
∠EAD = ∠ADF (EA || DF)
AD = AD (Common)
∴ ΔEAD `~=` ΔFDA (AAS congruence criterion)
⇒ EA = DF … (vi)
Adding equations (i) and (iii), we obtain
DA2 + AB2 + 2EA × AB + AD2 + DC2 − 2DC × FD = DB2 + AC2
DA2 + AB2 + AD2 + DC2 + 2EA × AB − 2DC × FD = DB2 + AC2
BC2 + AB2 + AD2 + DC2 + 2EA × AB − 2AB × EA = DB2 + AC2
[Using equations (iv) and (vi)]
AB2 + BC2 + CD2 + DA2 = AC2 + BD2
APPEARS IN
संबंधित प्रश्न
In a ∆ABC, AD ⊥ BC and AD2 = BC × CD. Prove ∆ABC is a right triangle
The perpendicular AD on the base BC of a ∆ABC intersects BC at D so that DB = 3 CD. Prove that `2"AB"^2 = 2"AC"^2 + "BC"^2`
In the given figure, AB//CD, AB = 7 cm, BD = 25 cm and CD = 17 cm;
find the length of side BC.
In the given figure, angle BAC = 90°, AC = 400 m, and AB = 300 m. Find the length of BC.

Find the Pythagorean triplet from among the following set of numbers.
4, 5, 6
In a triangle ABC right angled at C, P and Q are points of sides CA and CB respectively, which divide these sides the ratio 2 : 1.
Prove that: 9AQ2 = 9AC2 + 4BC2
Two trains leave a railway station at the same time. The first train travels due west and the second train due north. The first train travels at a speed of `(20 "km")/"hr"` and the second train travels at `(30 "km")/"hr"`. After 2 hours, what is the distance between them?
Sides AB and BE of a right triangle, right-angled at B are of lengths 16 cm and 8 cm respectively. The length of the side of largest square FDGB that can be inscribed in the triangle ABE is ______.

Two rectangles are congruent, if they have same ______ and ______.
Jiya walks 6 km due east and then 8 km due north. How far is she from her starting place?
