Advertisements
Advertisements
प्रश्न
The electric field in a region is given by `vec"E"` = 5 `hatk`N/C. Calculate the electric flux Through a square of side 10.0 cm in the following cases
- The square is along the XY plane
- The square is along XZ plane
- The normal to the square makes an angle of 45° with the Z axis.
उत्तर
Given: `vec"E"` = 5 `hatk` N/C, |E| = 5 N/C, l = 10 cm = 10 × 10−2 m = 10−1 m, A = l2 = 10−2m2
To find: Electric flux in three cases (Φ1), (Φ2), (Φ3)
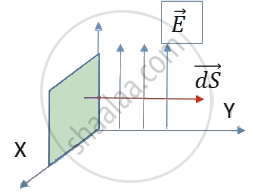
(i) Given the plane of Area is along XY plane. hence the direction of its normal `(vec"dS")` vector will be along Z axis.

The Angle between `vec"E"` and `"d"vec"S"` is zero i.e. θ = 0°.
∴ Φ = EA cos θ
∴ Φ1 = 5 × 10−2 cos 0
∴ Φ1 = 5 × 10−2 V m
(ii) Plane of Area is along XZ plane. Hence the direction of its normal `(vec"dS")` vector will be along Y axis.
∴ θ = 90°
∴ Φ = EA cos θ
∴ Φ2 = 5 × 10−2 cos 90°
∴ Φ2 = 0 V m
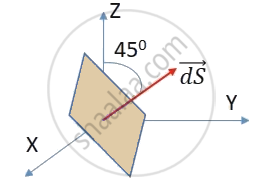
(iii) When normal to the square makes an angle of 45° with the Z axis.
∴ θ = 45°
∴ Φ = EA cos θ
∴ Φ3 = 5 × 10−2 cos 45°
∴ Φ3 = 3.5 × 10−2 V m
Electric flux in the above-mentioned cases are 5 × 10−2 V m, 0 V m, and 3.5 × 10−2 V m.
APPEARS IN
संबंधित प्रश्न
How does the electric flux due to a point charge enclosed by a spherical Gaussian surface get affected when its radius is increased?
Write its (electric flux.) S.I unit.
Given a uniform electric field `vecE=5xx10^3hati`N/C, find the flux of this field through a square of 10 cm on a side whose plane is parallel to the y-z plane. What would be the flux through the same square if the plane makes a 30° angle with the x-axis ?
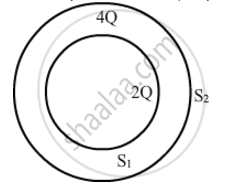
Consider two hollow concentric spheres, S1 and S2, enclosing charges 2Q and 4Q respectively as shown in the figure. (i) Find out the ratio of the electric flux through them. (ii) How will the electric flux through the sphere S1 change if a medium of dielectric constant 'εr' is introduced in the space inside S1 in place of air ? Deduce the necessary expression
Consider a uniform electric field E = 3 × 103 `bbhat i` N/C.
- What is the flux of this field through a square of 10 cm on a side whose plane is parallel to the yz plane?
- What is the flux through the same square if the normal to its plane makes a 60° angle with the x-axis?
Careful measurement of the electric field at the surface of a black box indicates that the net outward flux through the surface of the box is 8.0 × 103 N m2/C.
- What is the net charge inside the box?
- If the net outward flux through the surface of the box were zero, could you conclude that there were no charges inside the box? Why or Why not?
A uniformly charged conducting sphere of 2.4 m diameter has a surface charge density of 80.0 μC/m2.
- Find the charge on the sphere.
- What is the total electric flux leaving the surface of the sphere?
Given a uniform electric field \[\vec{E} = 2 \times {10}^3 \ \hat{i}\] N/C, find the flux of this field through a square of side 20 cm, whose plane is parallel to the y−z plane. What would be the flux through the same square, if the plane makes an angle of 30° with the x−axis ?
Given a uniform electric filed \[\vec{E} = 4 \times {10}^3 \ \hat{i} N/C\]. Find the flux of this field through a square of 5 cm on a side whose plane is parallel to the Y-Z plane. What would be the flux through the same square if the plane makes a 30° angle with the x-axis?
Two charges of magnitudes −2Q and +Q are located at points (a, 0) and (4a, 0) respectively. What is the electric flux due to these charges through a sphere of radius ‘3a’ with its centre at the origin?
A thin straight infinitely long conducting wire having charge density λ is enclosed by a cylindrical surface of radius r and length l, its axis coinciding with the length of the wire. Find the expression for the electric flux through the surface of the cylinder.
A small plane area is rotated in an electric field. In which orientation of the area, is the flux of the electric field through the area maximum? In which orientation is it zero?
Mark the correct options:
If the flux of the electric field through a closed surface is zero,
(a) the electric field must be zero everywhere on the surface
(b) the electric field may be zero everywhere on the surface
(c) the charge inside the surface must be zero
(d) the charge in the vicinity of the surface must be zero
Electric charges are distributed in a small volume. The flux of the electric field through a spherical surface of radius 10 cm surrounding the total charge is 25 V m. The flux over a concentric sphere of radius 20 cm will be _____________ .
If the flux associated with a coil changes at the rate of 360 webers every 4 minutes, then the induced e.m.f. is ______
The SI unit of electric flux is ______.
A cylinder of radius R and length L is placed in a uniform electric field E parallel to the cylinder axis. The total flux for the surface of the cylinder is given by ______.
The electric field in a region is given by `bar"E" = 4hat"i" + 10hat"j"` N/C. The flux of this field through a square of 10 cm on a side whose plane is parallel to the XZ plane.
In a region of space having a uniform electric field E, a hemispherical bowl of radius r is placed. The electric flux Φ through the bowl is:
The S.I. unit of electric flux is ______
What will be the total flux through the faces of the cube (figure) with side of length a if a charge q is placed at

- A: a corner of the cube.
- B: mid-point of an edge of the cube.
- C: centre of a face of the cube.
- D: mid-point of B and C.
The electric charges are distributed in a small volume. The flux of the electric field through a spherical surface of radius 10 cm surrounding the total charge is 20 V-m. The flux over a concentric sphere of radius 20 cm will be ______.
A hollow sphere of radius R has a point charge Q at its centre. Electric flux emanating from it is `phi`. If both the charge and the radius of the sphere are doubled, electric flux emanating from the sphere will ______.
A hollow sphere of radius R has a point charge q at its centre. Electric flux emanating from the sphere is X. How will the electric flux change, if at all, when radius of the sphere is doubled?
